Contents
- Introduction
- Arrhenius Theory (Water system concept, 1884)
- Brønsted–Lowry Theory (Protonic concept, 1923)
- Lewis Theory (Electron pair acceptor – donor concept, 1923)
- General Properties of Acids
- General Properties of Bases
- Salts
- Hydrolysis of salts
- Amphoteric and Amphiprotic character
- Volumetric analysis of Acids and Bases
- Concentration of ions
- Self Dissociation / Auto - Ionization of Water
- [H+] and [OH–]
- Dissociation Constants of Acids and Bases (ka, kb)
- pH, pOH and pKw, pKa, pKb
- Dissociation of weak acids and weak bases
- Indicators
- Buffers and Henderson–Hasselbalch equation
- Summary
- Key Terms
- Recommended Videos
- Test Questions
- Discuss And Explain
Learning Objectives
By the end of this section, you should be able to:
- Explain Arrhenius, Brønsted–Lowry, and Lewis concepts.
- Explain conjugate acid–base pair.
- Explain general properties of acids, bases and salts.
- Explain amphoterism.
- Explain Hydrogen ion and hydroxide ion concentration.
- Explain Molarity.
- Explain dissociation constants of acids/bases.
- Explain equilibrium of ionisation of weak acids/bases.
- Explain potential Hydrogen.
- Explain Buffers and Henderson–Hasselbalch equation.
Introduction
I believe that this is not your first time to hear the words acids, bases and salts. You must have discussed before now, the different types of acids, i.e, mineral/organic acids, several organic acids and their sources, like citric acid from lime/lemon, ethanoic acid from vinegar, weak/strong acids and bases, and also how salt is formed by neutralisation reaction between an acid and a base. This section will discuss a bit on all of these, and at the same time, expose you more to the chemistry of acids, bases and salts.
Acids, bases and salts are so wide that they do not have a specific definition, because every proposed definition has limitations, so we cannot really define acids and bases, but we can discuss the different theories that describe acids and bases. There are many theories, but only three are the most recognised.
Arrhenius Theory (Water system concept, 1884)
Svante August Arrhenius (1859 - 1927) is a Swedish Scientist who proposed a definition for acids and bases in his Doctoral Thesis in 1884.
Arrhenius defined acids as substances which dissociate (or ionize, in layman words= to chemically separate into ions) in a solvent to produce Hydrogen ions (H+ ions), while bases are substances that dissociate in a solvent to produce hydroxide ions (OH–).
For example:
$$\textnormal{HCl} \to \textnormal{H}^+ + \textnormal{Cl}^-$$
$$\textnormal{NaOH} \to \textnormal{Na}^+ + \textnormal {OH}^-$$
HCl is an acid because it dissociates to produce Hydrogen ion.
NaOH is a base because it dissociates to produce hydroxide ion.
However, chemists later realised that hydrogen ion (H+) cannot exist alone in aqueous solution, it exists in a combined state with a water molecule. The combination of hydrogen ion and a water molecule form hydroxonium ion (H3O+).
The dissociation of acids in water solvent is then written more accurately as:
$$\textnormal{HCl} + \textnormal{H}_2\textnormal{O} \to \textnormal{H}_3\textnormal{O}^+ + \textnormal{Cl}^-$$
Arrhenius' definition for acids and bases was not accepted in 1884, and Arrhenius barely received a passing mark on his work, but things changed about 20 years later when he got awarded with a Nobel prize in chemistry for the work reported in his thesis.
Arrhenius Theory, though it is accepted, still it has major flaws and limitations which opened way for more theories.
Applications (or Advantages) of Arrhenius Theory
• Arrhenius' Theory made us to see that oxides of non-metals (e.g CO2, SO2, SO3, N2O3, N2O5, P4O6, P4O10 etc) are acidic in nature, because they give H+ ions when they dissolve in water.
$$\small { \textnormal{SO}_3 + \textnormal{H}_2\textnormal{O} ⇆ \textnormal{H}_2\textnormal{SO}_4 ⇆ 2\textnormal{H}^+ + \textnormal{SO}_4^{2-}}$$
$$\small { \textnormal{N}_2\textnormal{O}_5 + \textnormal{H}_2\textnormal{O} ⇆ 2\textnormal{HNO}_3 ⇆ 2\textnormal{H}^+ + \textnormal{NO}_3^{2-}}$$
• Arrhenius' Theory made us to see that oxides of metals (e.g CaO, Na2O etc) and compounds like NH3, N2H4, NH4OH etc, are basic in nature, since these substances give OH– ions when they dissolve in water.
$$\small { \textnormal{CaO} + \textnormal{H}_2\textnormal{O} ⇆ \textnormal{Ca(OH)}_2 ⇆ \textnormal{Ca}^{2+} + 2\textnormal {OH}^-}$$
$$\small { \textnormal{NH}_3 + \textnormal{H}_2\textnormal{O} ⇆ \textnormal{NH}_4\textnormal{OH} ⇆ \textnormal{NH}_4^{+} + \textnormal {OH}^-}$$
• The catalytic property of acids in many reactions can be explained as due to the presence of H+ ions which are readily available from acids in aqueous medium.
Limitations of Arrhenius Theory
• According to Arrhenius concept, the acidic nature of a substance is only dependent on its aqueous solution. This means that an acid is not an acid unless it dissolves in a solvent to produce H+ ions. For example, HCl is considered as an acid only when it is dissolved in water, but it is not considered as an acid in its gaseous state or in another solvent in which it cannot produce H+ ions.
• Arrhenius Theory explains neutralisation reactions taking place only in a water medium, but cannot explain the reactions that occur in other non-aqueous solvents or in the gaseous phase. For example, the formation of \(\textnormal {NH}_4\textnormal{Cl}_{(s)}\) by the combination of \(\textnormal{NH}_{3(g)}\) and \(\textnormal{HCl}_{(g)}\) cannot be explained by Arrhenius concept.
$$\textnormal{NH}_{3(g)} + \textnormal{HCl}_{(g)} ⇆ \textnormal {NH}_4\textnormal{Cl}_{(s)}$$
Brønsted–Lowry Theory (Protonic concept, 1923)
Johannes Nicholar Brønsted (1879 - 1947) and Thomas Martin Lowry (1874 - 1936) are two contemporary scientists who were not working together, but they both developed relatively same and complementary definition of acids and bases.
According to Brønsted and Lowry, an acid is any substance that is capable of donating a proton to another substance, while a base is any substance that is capable of accepting a proton from any other substance.
For example:
$$\textnormal{HCl} + \textnormal{NH}_3 ⇆ \textnormal{NH}_4^+ + \textnormal{Cl}^-$$
In the above equation, HCl acts as an acid by donating a proton which is H+, while NH3 acts as a base by accepting the proton that has been donated by HCl, forming NH4+.
Another example is:
$$\textnormal{CH}_3\textnormal{COOH} + \textnormal{H}_2\textnormal{O} ⇆ \textnormal{CH}_3\textnormal{COO}^- + \textnormal{H}_3\textnormal{O}^+$$
In the above equation, CH3COOH acts as an acid by donating a proton which is H+, to become CH3COO–, while H2O acts as a base by accepting the proton that has been donated by CH3COOH, forming H3O+.
A Brønsted–Lowry acid–base reaction can proceed in both forward and backward directions. Something interesting now happens:
Using the above equation of reaction as an instance, Ethanoic acid (CH3COOH) donates a proton (H+) to a water molecule in the forward reaction, thus ethanoic acid acts as the acid (=proton donor) while water is the base (=proton acceptor) in the forward reaction,
$$\textnormal{CH}_3\textnormal{COOH} + \textnormal{H}_2\textnormal{O} \to \textnormal{CH}_3\textnormal{COO}^– + \textnormal{H}_3\textnormal{O}^+$$
But when we move backwards, in a backward reaction, what remained after ethanoic acid has lost a proton, which is ethanoate ion (CH3COO–) accepts a proton (back!) from the hydroxonium ion (H3O+) [that was formed when a proton attached to the base (water)],
This makes us to see that ethanoate ion is acting as the base (=proton acceptor) while hydroxonium ion is acting as the acid (=proton donor) in the backward reaction.
$$\textnormal{CH}_3\textnormal{COOH} + \textnormal{H}_2\textnormal{O} ←\textnormal{CH}_3\textnormal{COO}^- + \textnormal{H}_3\textnormal{O}^+$$
This entire scenario then leads us to say that when a substance donates a proton to become an acid in a forward reaction, what is left can also try to accept back protons in the backward reaction, making it a base, this type of base is called Conjugate base (of the acid).
On the other hand, a substance that acts as a base by accepting proton in the forward reaction, what is formed can also try to donate the proton which it has gotten, making it an acid (because it is donating protons) in the backward reaction, this type of acid is called Conjugate acid (of the base).
In summary;
What remains after an acid has donated a proton is called Conjugate Base.
What is formed when a base accepts a proton is called Conjugate acid.
This means that the both sides of every acid - base reaction (when we take Brønsted–Lowry concept into consideration) has an acid and a base.
An acid can only have a conjugate base. An acid will never have a conjugate acid, you should take note of that.
Also, a base can only have a conjugate acid, and never a conjugate base.
This is why we can only have conjugate acid - base pair, and never conjugate acid - acid pair or conjugate base - base pair, those are abominations!
PRO TIP: Conjugate means to be directly related, forming a pair.
| SPECIE | CONJUGATE BASE |
|---|---|
| CH3COOH | CH3COO- |
| H2O | OH- |
| NH3 | NH2- |
| HNO3 | NO3- |
| H2SO4 | HSO4- |
| H3PO4 | H2PO4- |
| SPECIE | CONJUGATE ACID |
|---|---|
| NH3 | NH4+ |
| H2O | H3O+ |
| OH- | H2O |
| H2NCONH2 (urea) | H2NCONH3+ |
EXAMPLE 1:
(a) HSO3– is the conjugate acid of which specie?
(b) HSO3– is the conjugate base of which specie?
Solution
(a)
Since conjugate acid is what is formed when a specie accepts a proton, HSO3– is the conjugate acid of SO32–.
When we remove a proton (H+) from HSO3–, what we have left is SO32– which is the original specie.
(b)
A conjugate base is what remains after a specie loses a proton, hence, when a particular specie lost a proton, HSO3– remained, adding back the lost proton (H+) to HSO3–, we have H2SO3.
HSO3– is the conjugate base of H2SO3.
EXAMPLE 2:
(a) Write an equation in which HSO4– reacts (with water) to form its conjugate base.
(b) Write an equation in which HSO4– reacts (with water) to form its conjugate acid.
Solution
(a)
For a conjugate base to be formed, HSO4– will have to act as an acid by donating a proton. Our equation can then be written as:
$$\small { \textnormal{HSO}_4^- + \textnormal{H}_2\textnormal{O} ⇆ \textnormal {SO}_4^{2-} + \textnormal{H}_3\textnormal{O}^+}$$
(b)
HSO4– acts as a base by gaining a proton to form its conjugate acid, the equation for the reaction is:
$$\small { \textnormal{HSO}_4^- + \textnormal{H}_2\textnormal{O} ⇆ \textnormal{H}_2\textnormal{SO}_4 + \textnormal{OH}^–}$$
When we take several substances into consideration with respect to Brønsted–Lowry Theory, we are able to classify Brønsted–Lowry Acids and Bases into:
Molecular acids and Molecular bases.
Anion acids and Anion bases.
Cation acids and Cation bases.
Molecular Acids
These are acids that basically possess neutral charge before donating a proton.
Examples are HNO3 (Nitric acid), H2SO4 (Sulphuric acid), HF (Hydrofluoric acid), CH3COOH (Acetic/Ethanoic acid) etc.
They donate a proton to give conjugate bases, which would normally be negatively charged ions.
$$\textnormal {HCl} \to \textnormal{Cl}^- + \textnormal{H}^+$$
$$\textnormal{H}_2\textnormal{SO}_4 \to \textnormal {HSO}_4^- + \textnormal{H}^+$$
$$\textnormal{CH}_3\textnormal{COOH} ⇆ \textnormal{CH}_3\textnormal{COO}^- + \textnormal{H}^+$$
Anion Acids
As the name describes, anions are negatively charged ions, therefore anion acids are acids that are in the form of negatively charged ions, but still have atleast one proton that they are able to donate.
Many of them are usually a result of polyprotic acids (=acids that have more than one proton that can be donated).
Examples of anion acids are HSO4– (Bisulphate), HSO3– (Bisulphite), HCO3– (Bicarbonate), H2PO4–, HC2O4– (Bi-oxalate).
$$\textnormal{HSO}_4^- ⇆ \textnormal{SO}_4^{2-} + \textnormal{H}^+$$
$$\textnormal{HC}_2\textnormal{O}_4^- ⇆ \textnormal{C}_2\textnormal{O}_4^{2-} + \textnormal{H}^+$$
Cation Acids
These are acids that are positively charged ions.
Examples are H3O+ (hydroxonium), NH4+ (ammonium), etc
$$\textnormal{H}_3\textnormal{O}^+ ⇆ \textnormal{H}_2\textnormal{O} + \textnormal{H}^+$$
$$\textnormal {NH}_4^+ ⇆ \textnormal{NH}_3 + \textnormal{H}^+$$
Molecular Bases
Just like molecular acids, molecular Bases are bases with no charge.
Examples are NH3 (ammonia), CH3NH2 (ethylamine), etc these bases gain protons to form cations.
$$\textnormal{NH}_3 + \textnormal{H}^+ ⇆ \textnormal{NH}_4^+$$
$$\textnormal {CH}_3\textnormal{NH}_2 + \textnormal{H}^+ ⇆ \textnormal{CH}_3\textnormal{NH}_3^+$$
Anion Bases
These are species that are negatively charged and can accept protons.
Examples are CO32–, SO42–, NO3-, S2-, Br-, Cl-, OH-, HSO4-, etc.
$$\textnormal{OH}^- + \textnormal{H}^+ ⇆ \textnormal{H}_2\textnormal{O}$$
$$\textnormal{CH}_3\textnormal{COO}^- + \textnormal {H}^+ ⇆ \textnormal{CH}_3\textnormal{COOH}$$
Cation Bases
These types of bases are rare, they are positively charged, but they still can accept more proton(s).
Examples are [Fe(H2O)5(OH)]2+, [Al(H2O)5(OH)]2+, etc
$$\small {\left [ \textnormal {Fe}(\textnormal{H}_2\textnormal{O})_5(\textnormal{OH})\right]^{2+} + \textnormal{H}^+ ⇆ \left[ \textnormal{Fe}(\textnormal{H}_2\textnormal{O})_6 \right]^{3+}}$$
| Type | Acid | Base |
|---|---|---|
| Molecular | HCl, HBr, HClO4, H2SO4, H3PO4, H2O | NH3, N2H4, amines, H2O |
| Anionic | HS–, HCO3–, HSO4–, H2PO4– | Cl–, Br–, OH–, HSO4–, CO32–, SO42– |
| Cationic | NH4+, H3O+, [Fe(H2O)6]3+, [Al(H2O)6]3+ | [Fe(H2O)5(OH)]2+, [Al(H2O)5(OH)]2+ |
Applications (or Advantages) of Brønsted–Lowry Theory
• Brønsted–Lowry concept explains the acidic/basic nature of a substance in aqueous (H2O) medium, and as well as in other Protonic solvents like liquid NH3, liq. HF, etc.
• This concept also explains acid-base reaction taking place in gaseous phase.
$$\small{\textnormal{HCl}_{(g)} + \textnormal {NH}_{3(g)} ⇆ \textnormal{NH}_4^+ + \textnormal {Cl}^– \: \: or \: \: \textnormal{NH}_4\textnormal{Cl}^-}$$
Limitations of Brønsted–Lowry Theory
• Brønsted–Lowry concept cannot explain acid - base reactions taking place in non-protonic substances, like liq. SO2, liq. BF3, BrF3, AlCl3, PoCl3, etc. in which no proton transfer takes place.
Lewis Theory (Electron pair acceptor – donor concept, 1923)
If you followed the previous topics that we discussed before now, you must have seen where it was said that some particular substances act as a Lewis acid.
Some substances do not have protons or hydrogen ions to donate, yet are still considered as acidic.
Gilbert Newton (G. N.) Lewis (1875 - 1946) established that acids are electron pair acceptors, while bases are electron pair donors.
In other words, Lewis defined acids as substances that can accept paired electrons, while bases are substances that have paired electrons which they can lose.
PRO TIP: accepting electron pair(s) by Lewis acids is different from losing a proton, although the net result is nearly the same= oxidation number decreases. Contrast is the case of losing electron pair.
We can also say that Lewis acids are substances that contain vacant orbitals (so that they can accept electron pairs) and Lewis bases are those substances that possess lone pair of electrons.
When a Lewis acid and a Lewis base undergo neutralisation reaction, they form a single compound which is called an Adduct or a Co-ordination Complex compound. These compounds usually contain (Lewis base–Lewis acid) Co-ordinate Covalent bond or Dative bond.
PRO TIP: Co-ordinate covalent bond is always represented by an arrow from the electron pair donor, to the electron pair acceptor.
Examples of Lewis acids are:
1. Molecules whose central atoms have vacant p orbital, or incomplete octet of electrons in its valence shell.
These molecules are usually those that fail to obey the octet rule. Common examples are BeF2, BH3, BF3, etc.
When we take a closer look at any of these, say BeF2, Beryllium, which is the central atom, has two electrons in its outermost 2s shell. These 2s electrons are paired and cannot bond, so, for Beryllium to form bonds, it becomes excited by unpairing its 2s electrons, promoting an electron into a 2p orbital. It then hybridizes and finally bonds with two atoms of Fluorine, withwhich the resulting covalent molecule is stable, despite Beryllium still having two vacant p orbitals. This molecule therefore, can accept electron pairs in its central atom's vacant p orbitals, and is said to be a Lewis acid.
Most simple covalent compounds in which Beryllium/Boron is the central atom, usually possess vacant p orbitals, making these compounds to act as Lewis acids.
2. Molecules whose central atoms have vacant d orbitals in their valence shells. Examples are molecules involving the transition elements and some p-block elements.
3. Any molecule in which its central atom is linked with more electronegative atom(s) by double bonds.
Examples are Carbon(IV) oxide (CO2) \(\left [\textnormal{O} = \textnormal{C} = \textnormal{O}\right]\), Sulphur(IV) oxide (SO2) \(\left [\textnormal{O} = \textnormal{S} = \textnormal{O}\right]\)
4. Simple cations. Normally, all cations can act as Lewis acids since they are electron deficient, but, cations such as Sodium ion (Na+), Potassium ion (K+), Calcium ion (Ca2+), etc., have a very little tendency to accept electrons. While cations like Silver ion (Ag2+), Copper ion (Cu2+), Cadmium ion (Cd2+), etc., have a greater tendency to accept electrons, therefore, they act as Lewis acids.
5. Elements that have 6 electrons in their valence shell (we say sextant of electrons) can accept electron pair and therefore act as Lewis acids. E.g Oxygen, Sulphur, etc.
Examples of Lewis Bases are:
1. Molecules whose central atom have atleast one lone pair of electrons. Examples are NH3, amines (\(\textnormal {R} —\textnormal{NH}_2\)), alcohols (\(\textnormal{R} —\textnormal{OH}\)), etc.
2. Molecules containing \(\textnormal{C} = \textnormal{C}\) double bonds.
3. Halides, e.g CsF, CoCl2, XeCl4, XeF4, etc.
4. Anions, e.g CN– (Cyanide ion), Cl–, OH–, etc
Lewis acid–base theory does not affect the substances previously called Brønsted–Lowry bases, because any Brønsted–Lowry base must have a pair of non bonding electrons (lone pair) in order to accept a proton.
However, Lewis theory vastly expands the category previously called Brønsted–Lowry acids, notwithstanding, some brønsted–Lowry acids fail to bend to Lewis Theory.
A Chemist named Pearson, in 1963... classified all Lewis acids and bases into hard and soft acids and bases. He also included a third category called borderline acids and bases, wherein the acids and bases have characteristics that are intermediate between hard and soft acids/bases.
| Hard Acids | Soft Acids |
|---|---|
| d orbitals are either vacant or non-existent | Nearly full d orbitals |
| Smaller in size | Larger in size |
| These are mostly light metal ions generally associated with high positive oxidation state | These are mostly heavy metal ions generally associated with low positive (or even zero) oxidation state |
| Hard Acids | Borderline Acids | Soft Acids |
|---|---|---|
| H+, Li+, Na+, K+, Be2+, Ca2+, Sr2+, Mn2+, Al3+, Ga3+, In3+, La3+, Lu3+, Cr3+, Co3+, Fe3+, As3+, Si4+, Ti4+, U4+, Ce3+, Sn4+, VO2+, UO22+, MoO33+, BF3 | Fe2+, Co2+, Ni2+, Cu2+, Zn2+, Pb2+, Sn2+, Sb3+, Bi3+, Rh3+, NO+, SO2, GaH3 | Cu+, Ag+, Hg+, Au+, Tl+, Pb2+, Cd2+, Pt2+, Hg2+, Pt4+, Tl3+, BH3, GaCl3 InCl3, Carbenes, I+, Br+, O, Cl, Br, I, N, zero valent metal atoms |
| Hard Bases | Soft Bases |
|---|---|
| Electron pair Donor atoms having high electronegativity | Electron pair Donor atoms having low electronegativity |
| Hard Bases | Borderline Bases | Soft Bases |
|---|---|---|
| H2O, OH–, F–, CH3COO–, PO43–, SO42–, Cl–, CO32–, ClO4–, NO3–, ROH, RO–, R2O, NH3, NH2, N2H4 | C6H5NH2, C6H5N, N3–, NO2, SO32–, N2, Br– | R2S, RHS, RS–, I–, SCN–, S2O32-, R3P, R3As, CN–, RCN, CO, C2H4, C6H6, H– |
According to Hard and Soft Acids and Bases principle (HSAB principle), hard acids form stable complexes (adducts) with hard bases, and soft acids with soft bases.
Applications (or Advantages) of Lewis Theory
• Lewis Theory includes those reactions in which no protons are involved.
• Lewis Theory is more significant than Brønsted–Lowry concept because according to Lewis concept, acid-base behaviour is independent of solvent's presence or absence.
• Lewis concept further explains the basic properties of metallic oxides and acidic properties of non-metallic oxides.
Limitations of Lewis Theory
• Protonic acids like H2SO4 and HCl are not covered under Lewis concept because they do not establish a covalent bond by accepting a pair of electron (which a Lewis acid ought to).
Summary of the Concepts
| Concept | Acid | Base | Product |
|---|---|---|---|
| Arrhenius | Gives H+/H3O+ | Gives OH– | Salt + H2O |
| Brønsted - Lowry | Donates proton (H+) | Accepts proton (H+) | Conjugate Base + Conjugate Acid |
| Lewis | Accepts Electron pair | Donates Electron pair | Adduct |
It is note-worthy that:
All Arrhenius acids and bases are also Brønsted–Lowry acids and bases.
But, not all Brønsted–Lowry acids and bases are Arrhenius acids and bases.
Brønsted–Lowry bases are also Lewis bases, but not all Brønsted–Lowry acids are Lewis acids.
Not all Lewis acids and bases are also Brønsted–Lowry acids and bases.
Other acid–base concepts are Ingold Robinson Theory (Electrophile/Electron pair acceptor - Nucleophile/Electron pair donor concept, 1932), Lux–Flood Theory (Oxide ion acceptor - Oxide ion donor concept, 1939), Usanovich Theory (Electron acceptor - Electron donor concept, 1939), Solvent System Concept (Solvent cation - solvent anion concept, 1950s), Frontier Orbitals Concept (LUMO of acceptor - HOMO of donor concept, 1960s).
General Properties of Acids
Physical Properties of Acids
• Acids usually have sour taste. Organic acids present in lemon, vinegar, curd, make them to taste sour. You should never taste acids in the Lab because that is very risky.
• Concentrated forms of acids are corrosive (= they attack various substances).
• Acids can conduct electric current in their aqueous solutions. This is due to their ability to dissociate into ions.
• Acids change the colour of blue litmus to red. Commonly used is the blue litmus paper which would turn red on contact with an acid.
PRO TIP: corrosive action of acids is not related to their strength (whether how strong or weak an acid is). It is related to the negatively charged part of the acid. For example, hydrofluoric acid, (HF) is a weak acid, yet, it is so corrosive that it attacks and dissolves even glass. This is due to the very high electronegativity of Fluorine. The fluoride ion attacks the silicon atom in the silicates in glass, while the hydrogen ion attacks the oxygen of silica (SiO2) in the glass.
Chemical Properties of Acids
Reaction with water
Concentrated acids react exothermically with water to form dilute acids, and like you all know, water is never poured into a concentrated acid unless you want to get burns as a result of violent splash, you should only pour concentrated acids into water, still never pour large quantities at once.
Acids dissociate into ions in water, and acids that dissociate completely (near 100%) in water are known as strong acids, while those which dissociate only partially (very much less than 100%) are known as weak acids.
The strongest weak acid is acetic/ethanoic acid (CH3COOH) and it dissociates approximately 1% in water.
$$\textnormal{HA} + \textnormal{H}_2\textnormal{O} \leftrightharpoons \textnormal{H}_3\textnormal{O}^+ + \textnormal{A}^-$$
Reaction with metals
Acids react with reactive metals to yield salt and hydrogen gas.
$$\textnormal{Zn}_{(s)} + 2\textnormal{HCl}_{(aq)} \to \textnormal{ZnCl}_{2(aq)} + \textnormal{H}_{2(g)}$$
$$2\textnormal{Na}_{(s)} + \textnormal{H}_2\textnormal{SO}_{4(aq)} \to \textnormal{Na}_2\textnormal{SO}_{4(aq)} + \textnormal{H}_{2(g)}$$
You should note that dilute HNO3 is an exception to this rule. Water is produced, instead of hydrogen gas.
$$\small {2\textnormal{HNO}_3 + \textnormal{Na} \to \textnormal{NaNO}_3 + \textnormal{NO}_2 + \textnormal{H}_2\textnormal{O}}$$
Reaction with Carbonates and Bicarbonates
Acids react with Carbonates and Bicarbonates to form corresponding salts and with the evolution of Carbon(IV) oxide gas.
$$\scriptsize {\textnormal{CaCO}_{3(s)} + \textnormal{H}_2\textnormal{SO}_{4(aq)} \to \textnormal{CaSO}_{4(aq)} + \textnormal{H}_2\textnormal{O}_{(l)} + \textnormal{CO}_{2(g)}}$$
$$ \scriptsize {\textnormal{NaHCO}_{3(s)} + \textnormal{HNO}_3 \to \textnormal{NaNO}_{3(aq)} + \textnormal{H}_2\textnormal{O} + \textnormal{CO}_{2(g)}}$$
Reaction with Sulphites and Bisulphites
Acids react with Sulphites and Bisulphites to form salts with the liberation of sulphur(IV) oxide gas.
$$\scriptsize {\textnormal{CaSO}_{3(aq)} + 2\textnormal{HCl} \to \textnormal{CaCl}_{2(aq)} + \textnormal{H}_2\textnormal{O}_{(l)} + \textnormal {SO}_{2(g)}}$$
$$\scriptsize {\textnormal{NaHSO}_{3(aq)} + \textnormal{HCl}_{(aq)} \to \textnormal{NaCl}_{(aq)} + \textnormal {H}_2\textnormal{O}_{(l)} + \textnormal{SO}_{2(g)}}$$
Reaction with Bases
Acids react with bases (oxides and hydroxides of metal, ammonium hydroxide) to form salts and water. This is a neutralisation reaction.
$$\small {\textnormal{NaOH}_{(s)} + \textnormal{HCl}_{(aq)} \to \textnormal{NaCl}_{(aq)} + \textnormal{H}_2\textnormal{O}_{(l)}}$$
$$\small {\textnormal {Al}_2\textnormal{O}_{3(s)} + 3\textnormal{H}_2\textnormal{SO}_{4(aq)} \to \textnormal {Al}_2(\textnormal{SO}_4)_{3(aq)} + 3\textnormal{H}_2\textnormal{O}_{(l)}}$$
Reaction with Sulphides
Acids react with metal sulphides to liberate a foul smelling gas called hydrogen sulphide gas.
$$\small {\textnormal{FeS}_{(s)} + \textnormal{H}_2\textnormal{SO}_{4(aq)} \to \textnormal{FeSO}_{4(aq)} + \textnormal{H}_2\textnormal{S}_{(g)}}$$
Basicity of an Acid
The basicity of an acid is simply the number of replaceable hydrogen atoms that is possessed by an acid.
In other words, basicity of an acid is a term that describes how many hydrogen atoms, present in an acid, can be replaced by metal.
Monobasic acids have only one hydrogen atom which can be replaced by metal. Examples are HCl, HNO3, HF, CH3COOH, etc. These monobasic acids can only donate one hydrogen atom, and they are sometimes called Monoprotic. Monoprotic acids are acids that can donate only one proton (they systematically extend beyond only monobasic acids).
$$\textnormal{HCl} \to \textnormal{H}^+ + \textnormal{Cl}^-$$
$$\textnormal{CH}_3\textnormal{COOH} ⇆ \textnormal{H}^+ + \textnormal{CH}_3\textnormal{COO}^-$$
You could see that not all the hydrogen atoms in CH3COOH are replaceable. All carboxylic acids (R —COOH) are monobasic.
Dibasic acids have two replaceable hydrogen atoms. Examples are H2SO4, H2CO3, H2SeO4 etc.
Polybasic acids have several hydrogen atoms that can be replaced by metals. An example is H3PO4. They are also referred to as polyprotic because they can donate several protons.
Non-protic acids have no proton (or hydrogen atoms) that they can donate, most of them act as Lewis acids.
Acids and Conjugate Bases
Brønsted–Lowry concept helped us to see that when acids lose proton (to a base) in a forward reaction, what remains can undergo a reverse reaction by accepting a proton, thus, becoming a base that is called Conjugate base (of the acid).
It is noteworthy that when a strong acid loses a proton to give rise to a conjugate base, the conjugate base interacts less with proton(s), and thus hardly forms back the strong acid, it is therefore said that strong acids have weak conjugate bases.
In other words, the ionization of strong acids are usually written as only forward reactions, because usually at the end, very little to no concentration of the original acid will be left, only its dissociated ions will remain in the solution which cannot form back the acid. Thus, dissociation of strong acids is not an equilibrium reaction. (equilibrium reaction=reaction which does not reach completion under normal conditions, in which the products can form back the reactants).
On the other hand, weak acids partly ionize, and when they lose a proton to give rise to a conjugate base, the conjugate base can interact strongly (with proton, in a reverse reaction) to form back the weak acid, hence, it is said that weak acids have strong conjugate bases.
The stronger the acid, the weaker its conjugate base!
| Strong Acids | Weak Acids |
|---|---|
| The acids which dissociate completely in water are called strong acids | The acids which dissociate partially in water are called weak acids |
| Strong acids are commonly mineral acids | All organic acids and some inorganic acids are weak acids |
| Since dissociation of strong acids is complete, the reaction is usually shown as a one sided arrow, the conjugate base of a strong acid cannot reform the strong acid in an aqueous solution | Since dissociation of weak acids is partial, the (dissociation) reaction is usually incomplete, and is depicted by double half arrows (or harpoons) |
| \(\scriptsize{\textnormal{HNO}_3 \to \textnormal{H}^+ + \textnormal{NO}_3^-}\) | \(\scriptsize{\textnormal{HF} \rightleftharpoons \textnormal{H}^+ + \textnormal{F}^-}\) |
| Little to no concentration of a strong acid is left in its aqueous solution | Aqueous solutions of weak acids contain concentrations of the dissociated ions and also the undissociated acid |
| There's usually no equilibrium between a strong acid and its dissociated ions in an aqueous solution, because the dissociation reaction is complete | Dissociation reaction (into ions) in aqueous solutions never reaches completion, eventually, equilibrium is achieved between the (undissociated) acid and the dissociated ions |
|
There are only seven known strong acids: HCl (Hydrochloric Acid) HBr (Hydrobromic Acid) HI (Hydroiodic Acid) HClO3 (Chloric Acid) HClO4 (Perchloric Acid) HNO3 (Nitric Acid) H2SO4 (Sulphuric Acid) |
Examples of Weak Acids are: CH3COOH (Acetic/Ethanoic Acid) HF (Hydrofluoric Acid) HCN (Hydrocyanic Acid) HCOOH (Formic Acid) H2CO3 (Carbonic Acid) C6H5COOH (Benzoic Acid) |
Inasmuch as I would have loved to talk about the superacids, it's out of our context, so I'll save it for another time.
Uses of Acids
• Hydrochloric acid is used for cleaning metals. If you put rust iron nail in HCl, after sometime, the iron nail would become as clean as new. If you try it with rust zinc sheet, the zinc will melt along with the rust, so, it is only metals that cannot displace hydrogen that are usually cleaned using this method. Sulphuric acid is also used for this purpose.
• Hydrochloric acid is also used in tanning industries and in printing industries.
• Nitric acid is used to make ammonium nitrate fertilisers.
• Nitric acid is also used in explosives, paints, drugs.
• Hydrofluoric acid is used to make design on glass. Stencils are usually used to cover the parts that the acid should not touch, the acid then melts the exposed parts, etching designs and patterns.
• Sulphuric acid is usually used in the lab as a dehydrating agent.
• Sulphuric acid is also used to make dyes, paints, fertilisers, drugs, and it also serve as an electrolyte in lead-acid batteries. Sulphuric acid is usually called King of acids because it is the most produced acid in the world and it has very wide range of uses.
• Benzoic acid is used for food preservation.
• Acetic acid is also used for food preservation, and food flavouring.
General Properties of Bases
Physical Properties of Bases
• Bases are said to have bitter taste. Never taste a base in the lab!
• Bases have soapy feel. An example is caustic soda which you must have seen in soap making practicals, or potash (káun) that we normally use during cooking of jute leaf (ewédú) and okra, and to make potash fertilisers. Again, never rub bases in the lab on your skin, say you want to experiment their soapy feel.
• Bases tend to be non-corrosive, except the concentrated forms of NaOH and KOH.
• The aqueous solutions of bases can conduct electric current.
• Bases change the colour of red litmus to blue.
• Not all bases are soluble in water. A base that is soluble in water is called an alkali, while the insoluble ones remain as base.
Chemical Properties of Bases
Reaction with water
Bases that react exothermically and dissolve in water are known as Alkalis. Alkalis that dissociate completely in water to give OH– ions and corresponding cations are called strong bases.
A few slightly-soluble bases are also strong bases (e.g calcium hydroxide, Strontium hydroxide), because the small percentage that dissolves in water dissociate completely into OH– ions and cations.
Weak bases on the other hand give lesser OH– ions in solution. Many cannot intrinsically give OH– ions, they have to first react with water before they can partly ionize to give hydroxide ions, an example is NH3.
Reaction with Acids
Bases react with acids to form salt and water.
$$\textnormal{KOH}_{(aq)} + \textnormal{HCl}_{(aq)} \to \textnormal{KCl}_{(aq)} + \textnormal{H}_2\textnormal{O}_{(l)}$$
Reaction with Ammonium salts
Bases react with Ammonium salts when heated together, to liberate ammonia gas.
$$\small {\textnormal{NH}_4\textnormal{Cl}_{(aq)} + \textnormal{NaOH}_{(aq)} \to \textnormal{NaCl}_{(aq)} + \textnormal{H}_2\textnormal{O}_{(l)} + \textnormal{NH}_3}$$
Bases and Conjugate Acids
According to Brønsted–Lowry concept, a base accepts proton from a proton donor (acid).
When a base has accepted proton, it forms a conjugate acid, which can in turn, donate the accepted proton in a reverse reaction.
Like acids, the stronger the base, the weaker the conjugate acid!
| Strong Bases | Weak Bases |
|---|---|
| Strong bases completely dissociate in water to give the cation and hydroxide ions (OH–) | Weak bases give lesser or do not give OH– ions by dissociation, they react with water to give OH– ions |
| \(\scriptsize{ \textnormal{KOH}\to \textnormal{K}^+ + \textnormal{OH}^-}\) | \(\tiny{\textnormal{NH}_3 + \textnormal{H}_2\textnormal{O} \to \textnormal{NH}_4\textnormal{OH} \rightleftharpoons \textnormal{NH}_4^+ + \textnormal{OH}^-}\) |
| Dissociation reaction reaches completion and there's no equilibrium achieved | Dissociation reaction does not reach completion, solutions contain relatively low concentration of OH– and equilibrium is achieved |
|
There are only 8 strong bases; the hydroxides of alkali metals and some alkaline earth metals LiOH (Lithium hydroxide) NaOH (Sodium hydroxide) KOH (Potassium hydroxide) RbOH (Rubidium hydroxide) CsOH (Caesium hydroxide) Ca(OH)2 (Calcium hydroxide) Sr(OH)2 (Strontium hydroxide) Ba(OH)2 (Barium hydroxide) |
Examples are NH4OH (Ammonium hydroxide) Cu(OH)2 (Copper hydroxide) Zn(OH)2 (Zinc hydroxide) etc. |
Uses of Bases
• NaOH is used in the manufacturing of soap. Soaps are produced from esters (or fatty acids) by saponification reaction with NaOH or KOH. Many say that Caustic soda (NaOH) is used for making washing soap, while potash (KOH) is used to make bathing soaps.
• Ca(OH)2 is used to manufacture bleaching powder (CaOCl2), cement, Plaster of Paris (CaSO4·\(\frac{1}{2}\)H2O), and also to neutralise acidic soil and lakes.
• Mg(OH)2 is a major component in milk of magnesia (MOM), used as an antacid (anti-acid), to neutralise excessive stomach acidity and heart burns that is as a result of back flow of HCl from the stomach into the oesophageal regions (or oesophagus).
• Mg(OH)2 is also usually used to neutralise formic (or methanoic) acid (HCOOH) that is injected by ants and bees when they sting.
• Mg(OH)2 is also used in toothpastes.
• (NH)4OH is used to remove grease stains in clothes.
• NaOH has very wide range of uses, and also in the production of other chemicals.
• KOH also has wide range of uses, used in fertilisers, alkaline batteries etc.
Salts
When salt is mentioned, what first comes into anyone's mind is table salt... range of salts extend far beyond just normal table salts. Salts are crystalline substances that are neither acid nor base.
Formation of Salts
Salts can be formed by neutralisation of acids and bases.
$$\textnormal{NaOH} + \textnormal{HCl} \to \textnormal{NaCl} + \textnormal{H}_2\textnormal{O}$$
$$\textnormal{KOH} + \textnormal{HNO}_3 \to \textnormal{KNO}_3 + \textnormal{H}_2\textnormal{O}$$
$$\textnormal{BOH} + \textnormal{HA} \to \textnormal{AB} + \textnormal{H}_2\textnormal{O}$$
The products of neutralisation reaction between an acid and a base are salt and water.
The positively charged cation of a salt (e.g \(\textnormal{Na}^+\) in \(\textnormal{Na}^+\textnormal{Cl}^-\)) comes from the base, and can be called Basic Radical; while the negatively charged anion of salts (e.g \(\textnormal{Cl}^-\) in \(\textnormal{Na}^+\textnormal{Cl}^-\)) comes from the acid, and can be called Acid Radical.
Salts can be formed by action of acids on metals
$$\textnormal{Zn} + 2\textnormal{HCl} \to \textnormal{ZnCl}_2 + \textnormal{H}_2$$
$$\textnormal{Fe} + \textnormal{H}_2\textnormal{SO}_4 \to \textnormal{FeSO}_4 + \textnormal{H}_2$$
Reactions between an acid and a reactive metal yield salt.
Salts can be formed by action of acids on carbonates and Bicarbonates
$$\scriptsize {\textnormal{CaCO}_{3(s)} + \textnormal{H}_2\textnormal{SO}_{4(aq)} \to \textnormal{CaSO}_{4(aq)} + \textnormal{H}_2\textnormal{O}_{(l)} + \textnormal{CO}_{2(g)}}$$
$$ \scriptsize {\textnormal{NaHCO}_{3(s)} + \textnormal{HNO}_3 \to \textnormal{NaNO}_{3(aq)} + \textnormal{H}_2\textnormal{O} + \textnormal{CO}_{2(g)}}$$
Types of Salts
Simple/Normal salts
These are chemically neutral salts. They are formed when all the replaceable hydrogen atoms that are present in an acid have been replaced by metal.
Examples are NaCl, KCl, CaSO4 etc.
Double salts
These are mixture of two different salts, stable in solid form, but would dissociate completely when in aqueous solution.
Double salts contain two different cations and usually one type of anion in one mixture.
Examples are CaCO3·MgCO3 (Dolomite), K2SO4·Al2(SO4)3·24H2O (Potash Alum), FeSO4·(NH4)2SO4·6H2O (Mohr's salt), KCl2·MgCl2·XH2O (Carnalite), etc.
Mixed Salts
These are salts that contain more than one basic or acid radicals. Examples are bleaching powder Ca(OCl)Cl (having two acid radicals), NaKCO3 (having two basic radicals).
Complex salts
These are mostly adducts that arise from reaction between Lewis acids and Lewis bases.
They are coordination compounds, composed of a central atom that is attached to by ligands.
They are composed of two (or more) different cations or anions, but unlike double salts, these complex salts hardly dissociate completely in aqueous solutions.
Examples are K4[Fe(CN)6], [Cu(NH3)4]SO4, K[Ag(CN)2], etc.
Acidic salts
People usually make a common misconception when defining acidic salts. Chemists define acidic salts as salts that still have replaceable hydrogen atoms present in them. In other words, acidic salts are formed when basic radicals (or cations) do not completely replace all the replaceable hydrogen atoms in an acid. Examples are NaHSO4, KHCO3, NaH2PO4. Acidic salts can usually react with bases to form normal salts and water.
$$\textnormal{H}_2\textnormal{SO}_4 + \textnormal{KOH} \to \textnormal{KHSO}_4 + \textnormal{H}_2\textnormal{O}$$
Potassium Bisulphate / Potassium hydrogen tetraoxosulphate(VI), \(\textnormal{KHSO}_4\), is the acidic salt that is formed by the incomplete replacement of the total replaceable hydrogen atoms in Sulphuric acid, by Potassium, this is because the base was not Stochiometrically enough.
It can further react with a base to produce a normal salt:
$$\textnormal{KHSO}_4 + \textnormal{KOH} \to \textnormal{K}_2\textnormal{SO}_4 + \textnormal{H}_2\textnormal{O}$$
Basic salts
These are salts that are formed by the incomplete neutralisation of a polyhydroxy base by an acid.
$$\small {\textnormal{Al(OH)}_3 + \textnormal{HCl} \to \textnormal{Al(OH)}_2\textnormal{Cl} + \textnormal{H}_2\textnormal{O}}$$
$$\small {\textnormal{Zn(OH)}_2 + \textnormal{HNO}_3 \to \textnormal{Zn(OH)NO}_3 + \textnormal{H}_2\textnormal{O}}$$
Hydrolysis of salts
Hydro = water
Lyse = breakdown
Hydrolysis of salts is simply the breakdown of salts in water.
When salts dissolve in water, they give solutions that are either neutral, acidic or basic.
Primarily, we test for a salt solution's acidity/basicity/neutrality by dipping red and blue litmus papers in the salt solutions.
Another method is making use of indicators.
Some observations have been carried out which are:
Salts that are made up of strong acids + strong bases, on dissolution in water, the solutions did not change the colour of red or blue litmus paper.
This means that these salts dissolved in water to give neutral solutions.
Examples are NaCl, KNO3, etc
On the other hand, salts that are made up of strong acids + weak bases, when dissolved in water, their solutions changed the colour of blue litmus paper to red, there was no action on red litmus paper.
We know that only acidic solutions can change the colour of blue litmus to red.
It was therefore concluded that salts composed of strong acid + weak base hydrolyse (or dissolve) in water to give solutions that are acidic.
Examples are Copper(II) sulphate salt (CuSO4), ammonium chloride salt (NH4Cl), Zinc sulphate salt (ZnSO4) etc.
$$\small { \textnormal{CuSO}_4 + 2\textnormal{H}_2\textnormal{O} \to \textnormal{Cu(OH)}_2 + \textnormal{H}_2\textnormal{SO}_4}$$
H2SO4 is a strong acid, all hydroxides that are not of alkali metals, and not of alkaline earth metals beneath magnesium (in group 2A of the periodic table), are weak bases.
$$\small { \textnormal{NH}_4\textnormal{Cl} + \textnormal{H}_2\textnormal{O} \to \textnormal{NH}_4\textnormal{OH} + \textnormal{HCl}}$$
$$\small { \textnormal{ZnSO}_4 + 2\textnormal{H}_2\textnormal{O} \to \textnormal{Zn(OH)}_2 + \textnormal{H}_2\textnormal{SO}_4}$$
When we took salts that are made up of weak acids + strong bases and dissolved them in water, and we dipped in blue litmus paper in the solution, there was no change in its colour, but when we dipped in red litmus paper, its red colour changed to blue, meaning that the solution was alkaline.
Examples of salts that hydrolyse to form alkaline solution are sodium carbonate (Na2CO3), sodium acetate (CH3COONa), potassium bicarbonate (KHCO3) etc.
$$\small {\textnormal{Na}_2\textnormal{CO}_3 + 2\textnormal{H}_2\textnormal{O} \to 2\textnormal{NaOH} + \textnormal{H}_2\textnormal{CO}_3}$$
$$\small {\textnormal{CH}_3\textnormal{COONa} + \textnormal{H}_2\textnormal{O} \to \textnormal{NaOH} + \textnormal{CH}_3\textnormal{COOH}}$$
The acidic and basic nature of salt solutions have been explained as due to the reaction between stronger conjugate acids/bases with water, forming back the respective weak base/acid, thus, leaving behind the weaker conjugate base/acid in the solution, which cannot form back the strong acid/base.
PRO TIP: everytime that I experience sudden stomach upset, I usually take Mist mag and the stomach ache disappears after few minutes. Mist mag contain Magnesium trisilicate salt (Mg2Si3O8··xH2O), sodium carbonate salt (Na2CO3), and sodium bicarbonate salt (NaHCO3), all of which dissolve in water to give basic solutions, which neutralise my stomach's hyperacidity that resulted into stomach ache. Hence, salts of weak acids + strong bases play important role in the production of antacids.
Amphoteric and Amphiprotic character
Some substances (especially oxides and hydroxides of some metals) can react with acids, and can also react with bases in aqueous solutions; such substances are said to be AMPHOTERIC.
Amphoteric substances have both acidic and basic properties, i.e, in the presence of an acid, an amphoteric substance will act as a base, and also in the presence of a base, an amphoteric substance will act as an acid.
Examples of amphoteric substances are water (H2O), Amino acids (COOH—R—NH2), Alumina/Aluminium(III) oxide (Al2O3), Beryllium oxide (BeO), Beryllium hydroxide (Be(OH)2), Gallium oxide (Ga2O3), Germanium(II) oxide (GeO), Germanium(IV) oxide (GeO2), Tin(II) oxide (SnO), Tin(IV) oxide (SnO2), Lead(II) oxide (PbO), Lead(IV) oxide (PbO2), Astatine(III) oxide (As2O3), Antimony(III) oxide (Sb2O3), Bismuth(III) oxide (Bi2O3), Aluminium hydroxide (Al(OH)3), Tin(II) hydroxide (Sn(OH)2), Lead(II) hydroxide (Pb(OH)2), Chromium(III) hydroxide (Cr(OH)3), Copper(II) hydroxide (Cu(OH)2), Zinc(II) hydroxide (Zn(OH)2), and Cadmium(II) hydroxide (Cd(OH)2).
Molecules or ions which can act as both Brønsted–Lowry acid and Brønsted–Lowry base are known as AMPHIPROTIC.
In other words, Amphiprotic substances can donate proton, and also gain proton.
The main difference between Amphiprotic substances and Amphoteric substances is that while an Amphiprotic substance can either accept and/or donate proton (depending on the condition it finds itself), an amphoteric substance acts more generally as an acid or a base, beyond just obeying Brønsted–Lowry theory.
Lewis acids, which can also act as a base are Amphoteric but not Amphiprotic.
All Amphiprotic substances are Brønsted–Lowry acids and bases, and are also Amphoteric substances, but not all Amphoteric substances are Amphiprotic.
Volumetric analysis of Acids and Bases
You must have carried out Acid-Base titration in the chemistry Lab during CHM 191 practicals in previous semester. That means you should already know that an acid–base titration is a laboratory procedure which involves the addition of measured volumes of an acid (or base) which has a known concentration, to measured volumes of a base (or acid) for the purpose of determining the unknown concentration of the base (or acid).
During acid–base titration, several parameters were considered in calculations:
Concentration of acid/base in grams per Litre (or grams per cubic decimetre, g/dm³). This is also called Mass Concentration.
Concentration of acid/base in Moles per Litre (or moles per cubic decimetre, mol/dm³, or Molar, M). This is called Molar Concentration.
Volume of Acid (VA)
Volume of Base (VB)
Stochiometric Number of moles of acid (nA)
Stochiometric Number of moles of base (nB)
Mass and Molar Mass of Acid and Base.
When we talk about concentration, concentration of a substance refers to how much of a substance is present in a given volume of a mixture/solution.
Concentration is always given as = \(\frac {\textnormal{amount}}{\textnormal{volume}}\)
Mass concentration of an acid/base is the amount (in grams) of an acid/base that is present in 1 Litre of a given solution.
In other words, Mass concentration of a substance can be said to be the ratio of the mass of the substance (that is found in its solution) to volume of the given solution (in dm³).
Mass concentration is usually given as:
Conc in g/dm³ = \(\frac{\textnormal{mass (in grams)}}{\textnormal{Volume (in dm³)}}\)
1000 cm³ (or mL) = 1 dm³ (or L), so the equation can also be written as
Conc in g/dm³ = \(\frac{\textnormal{mass (in grams)}}{\textnormal{Volume (in cm³)}} × \small {1000}\)
On the other hand, Molar Concentration of an acid/base is the amount (in moles) of an acid/base that is present in 1 Litre of a given solution.
In other words, Molar concentration of a substance can be said to be the ratio of the number of moles of a substance (that is found in its solution) to volume of given solution (in dm³).
Molar Concentration is given as:
Conc in mol/dm³ = \(\frac{\textnormal{Number of moles (in mol)}}{\textnormal{Volume (in dm³)}}\)
1000 cm³ = 1 dm³, so the equation can also be written as
Conc in mol/dm³ = \(\frac{\textnormal{Number of moles (in mol)}}{\textnormal{Volume (in cm³)}} × \small {1000}\)
When we substitute volume in both equations (for mass concentration and Molar concentration), we discovered that mass concentration and Molar concentration are related by the formula:
Mass Concentration = Molar Concentration × Molar Mass
In other words,
Concentration in g/dm³ = Concentration in mol/dm³ × Molar Mass in g/mol
The above formulas are used to calculate for the concentration of one substance in a solution.
At the end of every titration, when the concentration of either an acid/base is already known, and the titre values (volume) for the acid and bases have also been gotten, we get the unknown concentration of the base/acid by using the formula:
$$\frac{\textnormal{C}_A × \textnormal{V}_A}{\textnormal{C}_B × \textnormal{V}_B} = \frac {\textnormal{n}_A}{\textnormal{n}_B}$$
Where \(\textnormal{C}_A\) = Concentration of acid (in mol/dm³)
\(\textnormal{V}_A\) = Volume of acid
\(\textnormal{C}_B\) = Concentration of base (in mol/dm³)
\(\textnormal{V}_B\) = Volume of base
\(\textnormal{n}_A\) = Mole number of acid in a balanced equation of reaction
\(\textnormal{n}_B\) = Mole number of base in a balanced equation of reaction
We are basically going to be using these formulas in calculations.
Example 1
A solution contains 6.789 grams of H2SO3 in enough solvent to make 1000 mL of solution.
Calculate:
(a) Concentration of H2SO3 in g/dm³
(b) Concentration of H2SO3 in mol/dm³
(H=1, S=32, O=16)
Solution
Mass of H2SO3 = 6.789 grams
Volume of Solution = 1000 mL
We can only use volume in unit L or dm³, so we convert 1000 mL to Litres.
1000 mL = 1 L
(a)
Concentration in g/dm³ is also the mass concentration. It is equal to:
\(\frac {\textnormal{mass}}{\textnormal{volume}}\)
Substituting known values, we have:
\(\frac {6.789 \textnormal {g}}{1 \textnormal{L}}\)
\(\small {6.789 \textnormal{g/L}}\)
Concentration of H2SO3 in g/dm³ in the given solution is 6.789 g/dm³.
(b)
Concentration in mol/dm³ is also the molar concentration. It is equal to:
\(\frac {\textnormal{mole}}{\textnormal{volume}}\)
We do not have number of moles,
We can get number of moles by:
\(\frac {\textnormal{mass (in grams)}}{\textnormal{Molar mass (in g/mol)}}\)
However, we can use the second formula, to save time:
\(\small { \textnormal {Mass concentration}}\)
\(\small{ = \textnormal {Molar concentration × Molar mass}}\)
\(\small {\textnormal {Molar concentration}} = \frac {\textnormal{Mass concentration}}{\textnormal{Molar mass}}\)
Molar mass of H2SO3:
= (2 × 1) + (32) + (3 × 16)
= (2) + (32) + (48)
= 82 g/mol
We can therefore calculate for Molar concentration:
= \(\frac {6.789 \textnormal{g/dm³}}{82 \textnormal{g/mol}}\)
= \(\small {0.083 \textnormal{mol/dm³}}\)
Concentration of H2SO3 in mol/dm³ in the given solution is 0.083 mol/dm³.
Example 2
A solution contains 11.522 grams of KOH in enough water to make 350 mL of solution.
Calculate:
(a) Concentration of KOH in g/dm³
(b) Concentration of KOH in mol/dm³
(K=39, O=16, H=1)
Solution
Mass of KOH = 11.522 grams
Volume of solution = 350 mL
We can only use volume in unit L or dm³, so we convert 350 mL to dm³
If, 1000 mL = 1 dm³
Then, 350 mL = x dm³
Cross multiplying:
x × 1000 = 350 × 1
x = \(\frac{350}{1000}\)
x = 0.35 dm³
Volume of solution = 0.35 dm³
(a)
Concentration in g/dm³ is also the mass concentration. It is equal to:
\(\frac {\textnormal{mass}}{\textnormal{volume}}\)
Substituting known values, we have:
\(\frac {11.522}{0.35}\)
\(\small {32.92}\)
Concentration of KOH in g/dm³ in the given solution is 32.92 g/dm³
(b)
Concentration in mol/dm³ is given in:
\(\small { \textnormal {Mass concentration}}\)
\( \small{= \textnormal {Molar concentration × Molar mass}}\)
\(\small {\textnormal {Molar concentration}} = \frac {\textnormal{Mass concentration}}{\textnormal{Molar mass}}\)
But we do not know the Molar mass for KOH, we calculate that by:
(39) + (16) + (1)
= 56 g/mol
Molar concentration is then solved as:
= \(\frac {32.92 \textnormal{g/dm³}}{56 \textnormal{g/mol}}\)
= \(\small {0.589 \textnormal{mol/dm³}}\)
Concentration of KOH in mol/dm³ in the given solution is 0.589 mol/dm³.
Example 3
How many grams of HNO3 are required to prepare 500 mL of a 0.601 M HNO3 solution?
Solution
Volume = 500 mL
1000 mL = 1 L
500mL = x L
Cross multiplying,
1000 × x = 500 × 1
x = \(\frac{500}{1000}\)
x = 0.5 L
Therefore, Volume = 0.5 L
Molar concentration = 0.601 M
mass = ?
Recall,
Molar concentration = \(\frac{\textnormal{Number of moles}}{\textnormal{Volume}}\)
We calculate for number of moles;
\(\small { \textnormal{Number of moles}}\)
= Molar concentration \(\small × {\textnormal{Volume}}\)
\(\small { \textnormal{Number of moles}}\) = 0.601 × 0.5
\(\small { \textnormal{Number of moles}}\) = 0.3005 mol
Recall,
Number of moles = \(\frac{\textnormal {mass}}{\textnormal {Molar mass}}\)
We get Molar mass for HNO3 by:
(1) + (14) + (3 × 16)
(1) + (14) + (48)
63 g/mol
We can then Calculate for mass in grams:
\(\small {0.3005 \textnormal {mol}} = \frac{\textnormal {mass}}{63}\)
\(\small {0.3005 \textnormal {mol}} \xcancel{=} \frac{\textnormal {mass}}{63}\)
\(\small{ \textnormal {mass} = 0.3005 × 63}\)
\(\small{ \textnormal {mass} = 18.93}\) g
18.93 grams of HNO3 is required to prepare 500 mL of a 0.601 M HNO3 solution.
Example 4
25 cm³ of NaOH reacts completely with 23.7 cm³ of H2SO4 that has a concentration of 0.1 M. Calculate the concentration of NaOH in this reaction?
Solution
Volume of NaOH = 25 cm³
Volume of H2SO4 = 23.7 cm³
Concentration of H2SO4 = 0.1 M
Concentration of NaOH = ?
All given parameters are related by the equation:
$$\frac{\textnormal{C}_A × \textnormal{V}_A}{\textnormal{C}_B × \textnormal{V}_B} = \frac {\textnormal{n}_A}{\textnormal{n}_B}$$
\(\textnormal{C}_A\) is the concentration of the acid = 0.1 M
\(\textnormal{V}_A\) is the volume of the acid = 23.7 cm³
\(\textnormal{C}_B\) is the concentration of the base = unknown
\(\textnormal{V}_B\) is the volume of the base = 25 cm³
\(\textnormal{n}_A\) = number of moles of acid
\(\textnormal{n}_B\) = number of moles of base
We do not yet know \(\textnormal{n}_A\) and \(\textnormal{n}_B\) and to get these, we will need to write a balanced equation of reaction for the acid–base reaction:
$$\small { 2\textnormal{NaOH} + \textnormal{H}_2\textnormal{SO}_4 \to \textnormal{Na}_2\textnormal{SO}_4 + 2\textnormal{H}_2\textnormal{O}}$$
From the balanced equation of reaction, we now know:
\(\textnormal{n}_A\) = 1
\(\textnormal{n}_B\) = 2
Substituting all known values into the equation:
\(\frac {0.1 × 23.7}{\textnormal{C}_B × 25} = \frac{1}{2}\)
\(\frac {2.37}{\textnormal{C}_B × 25} = \frac{1}{2}\)
\(\frac {2.37}{\textnormal{C}_B × 25} \xcancel{=} \frac{1}{2}\)
\(\small { 2.37 × 2 = \textnormal{C}_B × 25 × 1}\)
\(\small {4.74 = \textnormal{C}_B × 25}\)
\(\small {\textnormal {C}_B × 25 = 4.74}\)
\(\frac {\textnormal{C}_B × 25}{25} = \frac{4.74}{25}\)
\(\frac {\textnormal{C}_B × \cancel {25}}{\cancel {25}} = \frac{4.74}{25}\)
\(\small {\textnormal{C}_B} = \frac{4.74}{25}\)
\(\small {\textnormal{C}_B} = \small {0.1896}\)
Thus, Concentration of NaOH in the given acid–base reaction is calculated as 0.19 M
Example 5
What is the Volume of a 0.1 M HCl solution containing 1.46 grams of HCl?
Solution
Molar concentration of HCl = 0.1 M
Mass of HCl = 1.46 g
Volume of HCl = ?
Recall,
Molar concentration = \(\frac{\textnormal{Number of moles}}{\textnormal{Volume}}\)
Number of moles = \(\frac{\textnormal {mass}}{\textnormal {Molar mass}}\)
We can calculate Molar mass of HCl by;
(1) + (35.5)
36.5 g/mol
Number of moles will then be:
= \(\frac {1.46}{36.5}\)
= 0.04 mol
We can then finally substitute our known values into the Molar concentration formula:
0.1 = \(\frac {0.04}{\textnormal {Volume}}\)
\(\textnormal{Volume}\) = \(\frac {0.04}{0.1}\)
\(\textnormal{Volume}\) = 0.4 dm³
1.46 grams of HCl is contained in 0.4 dm³ (or 400 mL) of a 0.1 M HCl solution.
Concentration of ions
We have just seen that concentration of a substance is the amount, of that substance, that is found in its solution. The same also applies to ions.
I will be staying within our context only, when acids/bases dissolve in a solvent (esp water), they dissociate into ions, you already know that.
Concentration of an ion is then simply said to be the amount of a particular ion that will be found in a given volume of a solution.
Concentration of ions are usually expressed in Molar (M) or mole per cubic decimetre (mol/dm³); which means that we are dealing with Molar concentration.
We have discussed earlier that when a strong acid/base dissociates into ions, it dissociates completely, (close to 100%), so that only its constituent ions are found in the solution, this makes calculations for the concentration of ions of strong acids/bases in a solution to be very easily carried out, which we're practically going to be doing right now.
But, before then, talking about a weak acid/base, they do not dissociate completely right? Yeah!
Solutions in which a weak acid is dissolved, contain both concentrations of the acid, and concentrations of its constituent ions (or dissociated ions). This is why their dissociation reaction is an equilibrium reaction, it never reaches completion. The same happens for weak bases.
Calculating for concentration of ions when a weak acid/base dissociates in water follow a different method which will be explained later in this post.
Let us delve into knowing how to calculate for concentration of ions in strong acids/bases solutions.
Example 1
Find the hydrogen ion concentration of HCl in 0.1 M hydrochloric acid solution.
Solution
This is very easy. I'll be analysing the steps to carry out in numbers:
Step 1: Write a balanced equation to show the dissociation of HCl into ions.
Since HCl is a strong acid, it ionizes completely in water:
$$\small { \textnormal{HCl} \to \textnormal{H}^+ + \textnormal{Cl}^-}$$
Note: note that we are using this type of equation just for convenience of calculation, the appropriate dissociation equation for acids is not written as this, you remember; hydrogen ions cannot exist alone in solutions.
Step 2: Get the number of moles of \(\textnormal{H}^+\) from the equation of reaction.
From the above equation of reaction, the mole number of \(\textnormal{H}^+\) = 1
Step 3: Get the Molar concentration of the acid.
The Molar concentration of HCl has been given in the question = 0.1 M
Step 4: Multiply the Molar concentration of the acid and the number of moles of \(\textnormal{H}^+\)
= 0.1 × 1
= 0.1
It is therefore calculated that the concentration of hydrogen ion in 0.1 M of HCl solution is 0.1 mol/dm³.
You see that it's a piece of cake, let us enter other ones.
Example 2
Find the hydrogen ion concentration in 0.04 M H2Y solution, assuming that H2Y is a strong acid.
(b) Find the concentration of the Y2– ions in the above solution.
Solution
Step 1: Write a balanced equation to show the dissociation of H2Y into ions.
Since H2Y is a strong acid, it ionizes completely in water:
$$\small {\textnormal{H}_2\textnormal{Y} \to 2\textnormal{H}^+ + \textnormal{Y}^{2–}}$$
Step 2: Get the number of moles of \(\textnormal{H}^+\) from the equation of reaction.
From the above equation of reaction, the mole number of \(\textnormal{H}^+\) = 2
Step 3: Get the Molar concentration of the acid.
The Molar concentration of the H2Y has been given in the question = 0.04 M
Step 4: Multiply the Molar concentration of the acid and the number of moles of \(\textnormal{H}^+\)
= 0.04 × 2
= 0.08 M
It is therefore calculated that the hydrogen ion concentration in 0.04 M of H2Y solution is 0.08 M.
(b)
We are told to find the concentration of Y2– ions. It follows exactly same processes as the main question, the only difference is that instead of calculating for hydrogen ion concentration, it is now Y2– ion concentration.
Step 1: Write a balanced equation to show the dissociation of H2Y into ions.
$$\small {\textnormal{H}_2\textnormal{Y} \to 2\textnormal{H}^+ + \textnormal{Y}^{2–}}$$
Step 2: Get the number of moles of Y2– from the equation of reaction.
From the above equation of reaction, the mole number of Y2– = 1
Step 3: Get the Molar concentration of the acid.
The Molar concentration of H2Y has been given in the question = 0.04 M
Step 4: Multiply the Molar concentration of the acid and the number of moles of Y2–
= 0.04 × 1
= 0.04 M
It is therefore calculated that the concentration of Y2– ion in 0.04 M of H2Y solution is 0.04 mol/dm³.
Example 3
Calculate the concentration of the constituent ions in 0.050 M HNO3 solution.
Solution
Step 1: Write a balanced equation to show the dissociation of HNO3 into ions.
$$\small {\textnormal{HNO}_3 \to \textnormal{H}^+ + \textnormal{NO}_3^–}$$
Step 2: Get the number of moles of the constituent ions from the equation of reaction.
mole number of H+ = 1
mole number of NO3– = 1
Step 3: Get the Molar concentration of the acid.
Molar concentration of the acid has been given in the question = 0.050 M
Step 4: Multiply the Molar concentration of the acid and the number of moles of the constituent ions.
For H+ = 0.050 × 1
= 0.050 M
For NO3– = 0.050 × 1
= 0.050 M
Therefore, concentration of hydrogen ion in 0.050 M nitric acid solution is 0.050 M, and concentration of nitrate ion is 0.050 M.
Example 4
Calculate the molar concentrations of Ba2+ and OH– ions in 0.030 M barium hydroxide solution.
Solution
Step 1: Write a balanced equation to show the dissociation of Ba(OH)2 into ions.
Ba(OH)2 is a strong base, it dissociates completely in water:
$$\small {\textnormal{Ba(OH)}_2 \to \textnormal{Ba}^{2+} + 2\textnormal{OH}^–}$$
Step 2: Get the number of moles of the constituent ions from the equation of reaction.
mole number of Ba2+ = 1
mole number of OH– = 2
Step 3: Get the Molar concentration of the base.
Molar concentration of Ba(OH)2 has been given in the question = 0.030 M
Step 4: Multiply the Molar concentration of the base and the number of moles of the constituent ions.
For Ba2+ = 0.030 × 1
= 0.030 M
For OH– = 0.030 M × 2
= 0.060 M
Therefore, concentration of barium ion in 0.030 M barium hydroxide solution is 0.030 M, and concentration of hydroxide ion is 0.060 M.
Example 5
Calculate the concentrations of the constituent ions in 75.8 g of CaCl2·6H2O in 8.00 L of solution.
Solution
Now this question is different from the ones that we have been solving, still we follow the same steps.
Step 1: Write a balanced equation to show the dissociation of CaCl2 into ions.
$$\small {\textnormal{CaCl}_2 \to \textnormal{Ca}^{2+}+ 2\textnormal{Cl}^–}$$
Step 2: Get the number of moles of the constituent ions from the equation of reaction.
mole number of Ca2+ = 1
mole number of Cl– = 2
Step 3: Get the Molar concentration of the salt.
Now, we were not given molar concentration in the question, but we were given mass and volume. We can calculate for Molar concentration.
Molar concentration = \(\frac{\textnormal{number of moles}}{\textnormal{volume}}\)
Recall,
number of mole = \(\frac{\textnormal{mass}}{\textnormal{molar mass}}\)
Hence, we have to find molar mass of the salt:
Molar mass of CaCl2·6H2O is:
= (40) + (2 × 35.5) + (6 × [ (2 × 1) + (16) ] )
= (40) + (71) + (6 × [ (2) + (16) ] )
= (111) + (6 × [18] )
= (111) + (108)
= 219 g/mol
number of mole will then be:
= \(\frac {75.8}{219}\)
= 0.34612 mol
We can then calculate Molar concentration as:
= \(\frac {0.34612}{8.00}\)
= 0.0433 M
Now that we know the Molarity of the salt, we can then proceed to next steps.
Step 4: Multiply the Molar concentration of the salt and the number of moles of the constituent ions.
For Ca2+ = 0.0433 × 1
= 0.0433 M
For Cl– = 0.0433 × 2
= 0.0866 M
Therefore, concentration of calcium ion in 8 L of a solution containing 75.8 g of Calcium chloride hexahydrate salt is 0.0433 M, and concentration of chloride ion is 0.0866 M.
Example 6
Calculate the concentrations of the constituent ions in the following solutions.
(a) 2.55 g of KOH in 1.50 L of solution.
(b) 0.720 g of Ba(OH)2 in 250 mL of solution.
(c) 1.64 g of Ca(NO3)2 in 100 mL of solution.
Solution
(a)
Step 1: Write a balanced equation to show the dissociation of KOH into ions.
$$\small {\textnormal{KOH} \to \textnormal{K}^+ + \textnormal{OH}^–}$$
Step 2: Get the number of moles of the constituent ions from the equation of reaction.
number of moles of K+ = 1
number of moles of OH– = 1
Step 3: Get the Molar concentration of the base.
We were not given molar concentration in the question, but we were given mass and volume. We can calculate for Molar concentration.
Molar concentration = \(\frac{\textnormal{number of moles}}{\textnormal{volume}}\)
Recall,
number of mole = \(\frac{\textnormal{mass}}{\textnormal{molar mass}}\)
Hence, we have to find molar mass of the KOH:
Molar mass of KOH is:
= (39) + (16) + (1)
= 56 g/mol
number of mole will then be:
= \(\frac {2.55}{56}\)
= 0.04554 mol
We can calculate Molar concentration as:
= \(\frac {0.04554}{1.5}\)
= 0.03 M
Now that we know the Molarity of the base, we can proceed to final step.
Step 4: Multiply the Molar concentration of the base and the number of moles of its constituent ions.
For K+ = 0.03 × 1
= 0.03 M
For OH– = 0.03 × 1
= 0.03 M
Therefore, concentration of potassium ion in 1.5 L of a solution containing 2.55 g of potassium hydroxide is 0.03 M, and concentration of hydroxide ion is 0.03 M.
(b)
Step 1: Write a balanced equation to show the dissociation of Ba(OH)2 into ions.
$$\small {\textnormal{Ba(OH)}_2 \to \textnormal{Ba}^{2+} + 2\textnormal{OH}^–}$$
Step 2: Get the number of moles of the constituent ions from the equation of reaction.
number of moles of Ba2+ = 1
number of moles of OH– = 2
Step 3: Get the Molar concentration of the base.
From the question, we only have:
0.720 g of Ba(OH)2
and 250 mL of solution
250 mL needs to be converted to Litre:
If, 1000 mL = 1 L
then, 250 mL = x L
Cross multiplying;
x × 1000 = 250
x = \(\frac{250}{1000}\)
x = 0.25 L
Volume is therefore, 0.25 L
We need to calculate for molar mass of Ba(OH)2 so as to get number of moles:
Molar mass of Ba(OH)2:
= (137) + ( 2 × [16 + 1] )
= (137) + (2 × [17] )
= (137) + (34)
= 171 g/mol
number of moles of Ba(OH)2:
= \(\frac{0.720}{171}\)
= 0.00421 mol
Molar concentration can then be given as:
= \(\frac {0.00421}{0.25}\)
= 0.01684 M
Now that we know the Molarity of Ba(OH)2, we can proceed to final step.
Step 4: Multiply the Molar concentration of Ba(OH)2 and the number of moles of its constituent ions.
For Ba2+ = 0.017 × 1
= 0.017 M
For OH– = 0.017 × 2
= 0.034 M
Therefore, concentration of Barium ion in 250 mL of a solution containing 0.720 g of barium hydroxide is 0.017 M, and concentration of hydroxide ion is 0.034 M.
(c)
Step 1: Write a balanced equation to show the dissociation of Ca(NO3)2 into ions.
$$\small {\textnormal{Ca}(\textnormal{NO}_3)_2 \to \textnormal{Ca}^{2+} + 2\textnormal{NO}_3^–}$$
Step 2: Get the number of moles of the constituent ions from the equation of reaction.
number of moles of Ca2+ = 1
number of moles of NO3– = 2
Step 3: Get the Molarity of Ca(NO3)2.
From the question, we only have:
1.64 g of Ca(NO3)2
and 100 mL of solution
100 mL needs to be converted to Litres:
If, 1000 mL = 1 L
then, 100 mL = x L
Cross multiplying;
x × 1000 = 100 × 1
x = \(\frac {100}{1000}\)
x = 0.1 L
Volume of solution is 0.1 L
We calculate for molar mass of Ca(NO3)2 next, so that we can get number of moles.
Molar mass of Ca(NO3)2:
= (40) + ( 2 × [ 14 + (3 × 16) ] )
= (40) + ( 2 × [ 14 + 48 ] )
= (40) + ( 2 × [62] )
= (40) + (124)
= 164 g/mol
We can then get number of moles as:
= \(\frac{1.64}{164}\)
= 0.01 mol
Molar concentration will finally be given as:
= \(\frac {0.01}{0.1}\)
= 0.1 M
Step 4: Multiply the Molar concentration of Ca(NO3)2 and the number of moles of the constituent ions.
For Ca2+ = 0.1 × 1
= 0.1 M
For NO3– = 0.1 × 2
= 0.2 M
Therefore, concentration of calcium ion in 100 mL of a solution containing 1.64 g of potassium hydroxide is 0.1 M, and concentration of nitrate ion is 0.2 M.
Example 7
Calculate the concentrations of the constituent ions in the following solutions.
(a) 18.4 g of HBr in 675 mL of solution.
(b) 1.77 g of Al2(SO4)3 in 400 mL of solution.
Solution
(a)
Step 1: Write a balanced equation to show the dissociation of HBr into ions.
$$\small {\textnormal{HBr} \to \textnormal{H}^+ + \textnormal{Br}^–}$$
Step 2: Get the number of moles of the constituent ions from the equation of reaction.
number of moles of H+ = 1
number of moles of Br– = 1
Step 3: Get the molar concentration of HBr.
From the question, we were only given
18.4 g of HBr
and 675 mL of solution.
675 mL, converted to Litres is:
1000 mL = 1 L
675 mL = x L
Cross multiplying;
1000 × x = 675 × 1
x = \(\frac{675}{1000}\)
x = 0.675 L
Volume is 0.675 L
To calculate for number of moles, we need molar mass of HBr,
Molar mass of HBr:
= (1) + (80)
= 81 g/mol
Now, we can calculate for number of moles as:
= \(\frac {18.4}{81}\)
= 0.2272 mol
We can finally calculate for molar concentration:
= \(\frac {0.2272}{0.675}\)
= 0.3365 M
Step 4: Multiply the Molar concentration of HBr and the number of moles of the constituent ions.
For H+ = 0.3365 × 1
= 0.3365 M
For Br– = 0.3365 × 1
= 0.3365 M
Therefore, concentration of hydrogen ion in 675 mL of a solution containing 18.4 g of hydrobromic acid is 0.3365 M, and concentration of bromide ion is 0.3365 M.
(b)
Step 1:
Write the ionisation equation for Al2(SO4)3:
$$\small { \textnormal{Al}_2(\textnormal{SO}_4)_3 \to 2\textnormal{Al}^{3+} + 3\textnormal{SO}_4^{2–}}$$
Step 2: write the number of moles of the constituent ions.
number of mole of Al3+ = 2
number of mole of SO42– = 3
Step 3: Find the Molarity of Al2(SO4)3.
Molar concentration of Al2(SO4)3 was not given in the question, but we can solve for it.
Given:
1.77 g, 400 mL.
Converting 400 mL to Litres;
1000 mL = 1 L
400 mL = x L
x × 1000 = 400 × 1
x = \(\frac {400}{1000}\)
x = 0.4 L
What's next is to calculate for the Molar mass of Al2(SO4)3:
= (2 × 27) + (3 × [ 32 + (4 × 16) ] )
= (54) + ( 3 × [ 32 + (64) ] )
= (54) + ( 3 × [96] )
= (54) + (288)
= 342 g/mol
Number of moles:
= \(\frac{1.77}{342}\)
= 0.0052 mol
Molar concentration:
= \(\frac {0.0052}{0.4}\)
= 0.013 M
Step 4: Multiplying molarity of Al2(SO4)3 and the mole number of its constituent ions.
For Al3+ = 0.013 × 2
= 0.026 M
For SO42– = 0.013 × 3
= 0.039 M
Therefore, concentration of aluminium ion in 400 mL of a solution containing 1.77 g of Aluminium sulphate is 0.026 M, and concentration of sulphate ion is 0.039 M.
Self Dissociation / Auto–Ionization of Water
We have learnt that water behave as an acid, and as a base, and it also helps in the dissociation of acids and bases. It is the most common protic solvent for acids and bases reactions.
One interesting fact about Water is that, Water can dissociate (into its constituent ions) in itself, and this is called self–dissociation or auto–ionization or autoprotolysis.
Self–dissociation of water occurs only to a very small degree; only about two out of every billion (109) water molecules are dissociated at room temperature, 25°C.
Equation for the self–dissociation of water is written as:
$$\small {\textnormal{H}_2\textnormal{O}_{(l)} + \textnormal{H}_2\textnormal{O}_{(l)} \rightleftharpoons \textnormal{H}_3\textnormal{O}^+_{(aq)} + \textnormal{OH}^–_{(aq)}}$$
Self-dissociation of water is an equilibrium reaction.
You must have known before now, that, concentration of the products of any equilibrium reaction, is directly related to the concentration of the reactants.
In other words, when:
$$\scriptsize{\textnormal{reactant A} + \textnormal{reactant B} \rightleftharpoons \textnormal{product C} + \textnormal{product D}}$$
Concentration of the reactants and products shown in the above equation are related by an equilibrium formula.
$$\scriptsize { k = \frac {[\textnormal{product C}] × [\textnormal{product D}]}{[\textnormal{reactant A}] × [\textnormal{reactant B}]}}$$
Where k = equilibrium constant.
Note: Concentration of anything is written as [anything].
Thus, when water undergo self-dissociation at room temperature;
$$\small {\textnormal{H}_2\textnormal{O}_{(l)} + \textnormal{H}_2\textnormal{O}_{(l)} \rightleftharpoons \textnormal{H}_3\textnormal{O}^+_{(aq)} + \textnormal{OH}^–_{(aq)}}$$
the relative concentrations of the products and the reactants are shown in an equilibrium equation as:
$$\small {k = \frac {[\textnormal {H}_3\textnormal{O}^+][\textnormal{OH}^-]}{[\textnormal{H}_2\textnormal{O}]^2}}$$
Where k = equilibrium constant.
Calculations show that pure liquid water has a molarity of 55 mol/dm³ at room temperature. However, this is usually overlooked because we make use of activity of water, which is theoretically shown to be 1, hence, the approximate concentration of pure water is 1 M.
Note: You should note that the activity of any pure substance in its standard state is defined to be unity (1).
Pure water is chemically neutral, and this means that concentration of Hydrogen ion, [H+], is equal to concentration of hydroxide ion, [OH–].
Concentration of Hydrogen ion in pure water is shown to be 1.0 × 10–7 mol/dm³ at room temperature.
Since this value is the same for [OH–], and activity of water [H2O] = 1; substituting known values into the equilibrium formula gives us:
$$\small {k} = \frac {[1.0 × 10^{–7}][1.0 × 10^{–7}]}{[1]^2}$$
$$\small {k = 1.0 × 10^{–14}}$$
This implies that equilibrium constant for the dissociation (or ionization) of water at room temperature is 1.0 × 10–14.
This constant is recognised worldwide as the ionisation constant of pure water, and k is written formally as kw.
Formula for kw is written better as:
$$\small {\textnormal{k}_w = [\textnormal{H}_3\textnormal{O}^+][\textnormal{OH}^–]}$$
$$ \small { 1.0 × 10^{-14} = [\textnormal{H}_3\textnormal{O}^+][\textnormal{OH}^–]}$$
It is noteworthy that dissociation constant of water changes with changes in temperature. Increase in temperature leads to a small increase in Dissociation constant, and vice versa.
Hydrogen ion Concentration [H+] / [H3O+] and Hydroxide ion concentration [OH–] of acids and bases
Whenever acids dissolve in a particular volume of water at room temperature to form a solution, concentration of H+ increases, while concentration of OH– decreases.
Product of the both concentrations must be equal to the ionisation constant of water.
In the case of bases; bases dissolve in water to increase concentration of OH–, thus reducing H+ concentration.
Product of the both concentrations must also be equal to the ionisation constant of water.
Dissociation Constants of Acids and Bases (Ka, Kb)
Strong acids dissociate completely in water while weak acids dissociate only to a small degree. The same occur for strong bases and weak bases.
When we look at an acid dissociation reaction:
$$\small {\textnormal{HA} + \textnormal{H}_2\textnormal{O} \rightleftharpoons \textnormal{H}_3\textnormal{O}^+ + \textnormal{A}^-}$$
It can be written in an equilibrium equation as:
$$\small { k =\frac{ [\textnormal{H}_3\textnormal{O}^+][\textnormal{A}^-]}{[\textnormal{HA}][\textnormal{H}_2\textnormal{O}]}}$$
Since in dilute solutions, [H2O] is independent of concentration and tends to unity, the equilibrium equation is re-written as:
$$\small { k =\frac{ [\textnormal{H}_3\textnormal{O}^+][\textnormal{A}^-]}{[\textnormal{HA}]}}$$
Where k = equilibrium constant of the acid.
This equation is used to analyse concentration of acids, and its ions in water,
however, considering the fact that when strong acids dissociate, there's little to no concentration of the original acid left, this means [HA] depletes, then the equilibrium equation for dissociation of strong acids in water is given as:
$$\small { k = [\textnormal{H}_3\textnormal{O}^+][\textnormal{A}^–]}$$
Equilibrium constant (k) for dissociation of acids in a particular solvent is given as ka.
The larger the acid dissociation constant, (= there's more concentration of H3O+ in the aqueous solution) the stronger the acid.
Conversely, considering the dissociation equation of a base:
$$\small {\textnormal{BOH} \rightleftharpoons \textnormal{B}^+ + \textnormal{OH}^-}$$
The equilibrium equation is written as:
$$ \small {k = \frac {[\textnormal{B}^+][\textnormal{OH}^–]}{[\textnormal{BOH}]}}$$
Since strong bases will undergo dissociation that is close to completion,
$$\small { k = [\textnormal{B}^+][\textnormal{OH}^–]}$$
Equilibrium constants (k) for dissociation of bases in a particular solvent is given as kb.
The larger the base dissociation constant, (= there's more concentration of OH– in the aqueous solution) the stronger the base.
It is mathematically shown that dissociation constants of acids and bases in a conjugate acid–base pair are related to the dissociation constant of water by:
$$\small {\textnormal{k}_a × \textnormal{k}_b = \textnormal{k}_w}$$
This means that there can only be relative concentrations of H3O+ and OH– in the same solution, at a particular temperature.
Under room temperature conditions;
$$\small {\textnormal{k}_a × \textnormal{k}_b = 1.0 × 10^{-14}}$$
A larger ka value = smaller kb value = [H3O+] > [OH–] = the solution is acidic.
A smaller ka value = larger kb value = [H3O+] < [OH–] = the solution is basic.
When ka = kb = the solution is neutral.
pH, pOH and pKw, pKa, pKb
You must have come across the term pH before now, because it is very commonly used.
Normally, concentration of Hydrogen ions, [H+], concentration of hydroxide ions, [OH–], ionisation constants of water, acids, and bases, kw, ka, and kb, are usually very small values.
These small values are not ideal for basic calculations most of the time, because they can even make one to get confused, therefore, a function p was introduced.
Many call the name of the function 'p' potency/potential, others call it power. Whichever one, is not cool, unless you know what p implies.
Anywhere you see p, p is simply the negative logarithm of what is being talked about/variable.
In other words, if I have a variable, M, then pM = –log M.
The essence of p is to transform very small values into larger, positive values. It does nothing more than that.
Note: log is in base 10 = log10. Base 10 is usually invisible in mathematical calculations.
Relative to our discussion:
If we have concentration of Hydrogen ion, [H+], then pH is given as:
pH = –log [H+]
If we have concentration of hydroxide ion, [OH–], then pOH is given as:
pOH = –log [OH–]
If we have ionisation constant of water, kw, then pKw is given as:
pKw = –log kw
If we have ionisation constant of an acid, ka, then pKa is given as:
pKa = –log ka
If we have ionisation constant of a base, kb, then pKb is given as:
pKb = –log kb
If we take the ionisation constant of water, kw, and calculate for pKw:
pKw = –log kw
We know that kw = 1.0 × 10–14
Then pKw = –log 1.0 × 10–14
pKw = 14
Thus, potential/power of the ionisation constant of water at room temperature is 14.
Furthermore, When we relate:
[H+][OH–] = kw
Taking the logarithm of both sides;
log ( [H+][OH–] ) = log kw
log [H+] + log [OH–] = log kw
Multiplying throughout by -1
–log [H+] + –log [OH–] = –log kw
Then;
pH + pOH = pKw
Thus;
$$\small {\textnormal{pH} + \textnormal{pOH} = 14}$$
For every acid/base solution, we only need anyone of all of these values, ([H+] or [OH–] or pH or pOH) and we can use it to calculate for all the others.
Let us take examples :)
Example 1
Calculate the concentrations of H3O+ and OH– ions in a 0.050 M HNO3 solution.
Solution
Step 1: write a balanced equation for the dissociation of HNO3 into ions.
The standard dissociation reaction is shown as:
$$\small {\textnormal{HNO}_3 + \textnormal{H}_2\textnormal{O} \to \textnormal{H}_3\textnormal{O}^+ + \textnormal{NO}_3^–}$$
Step 2: number of moles of H3O+ = 1 mol
Step 3: molar concentration of HNO3 = 0.050 M
Step 4: [H3O+] = 0.050 × 1mol
= 0.050 M
Now that we know concentration of H3O+ as 0.050 M, we can calculate for concentration of OH–:
Recall,
[H3O+][OH–] = 1.0 × 10–14
0.050 × [OH–] = 1.0 × 10–14
[OH–] = \(\frac {1.0 × 10^{–14}}{0.050}\)
[OH] = 2.0 × 10-13 M
Therefore, it is calculated that a 0.050 M of HNO3 solution contains 0.050 M hydrogen ion concentration, and 2 × 10–13 M hydroxide ion concentration.
A quick inference that can be made from above calculation is that since [H3O+] > [OH–], the solution is acidic.
Example 2
Calculate [H3O+], pH, [OH–], and pOH for a 0.015 M HNO3 solution.
Solution
Step 1: \(\textnormal{HNO}_3 + \textnormal{H}_2\textnormal{O} \to \textnormal{H}_3\textnormal{O}^+ + \textnormal{NO}_3^–\)
Step 2: number of mole of H3O+ = 1 mol
Step 3: molar concentration of acid = 0.015 M
Step 4: [H3O+] = 1 × 0.015
= 0.015 M
Now that we know [H3O+] as 0.015 M, we can calculate for the remaining unknown:
Calculating for [OH–]:
[H3O+][OH–] = kw
[OH–] = \(\frac {\textnormal{k}_w}{[\textnormal{H}_3\textnormal{O}^+]}\)
[OH–] = \(\frac {1.0 × 10^{-14}}{0.015}\)
[OH–] = 6.67 × 10-13 M
Calculating for pH:
pH = –log [H3O+]
pH = –log 0.015
pH = –(–1.82)
pH = 1.82
Calculating for pOH:
pH + pOH = 14
1.82 + pOH = 14
pOH = 14 – 1.82
pOH = 12.18
pOH can alternatively be gotten from [OH–] by –log [OH–]
[H3O+] = 0.015 M
[OH–] = 6.67 × 10–13 M
pH = 1.82
pOH = 12.18
Example 3
Calculate [H3O+], pH, [OH–], and pOH for a 0.015 M Ca(OH)2 solution.
Solution
Step 1: \(\textnormal{Ca(OH)}_2 \to \textnormal{Ca}^2 + 2\textnormal{OH}^–\)
Step 2: number of moles of OH– = 2
Step 3: molar concentration of Ca(OH)2 = 0.015 M
Step 4: [OH–] = 0.015 × 2
= 0.030 M
Now that we know concentration of OH– as 0.030 M, we can calculate for the remaining unknown.
Calculating for [H3O+]:
[H3O+][OH–] = kw
[H3O+] × 0.030 = 1 × 10–14
[H3O+] = \(\frac {1 × 10^{–14}}{0.030}\)
[H3O+] = 3.33 × 10–13 M
Calculating for pOH:
pOH = –log [OH–]
pOH = –log 0.030
pOH = –(–1.52)
pOH = 1.52
Calculating for pH:
pH + pOH = 14
pH + 1.52 = 14
pH = 14 – 1.52
pH = 12.48
[OH–] = 0.030 M
[H3O+] = 3.33 × 10–13 M
pOH = 1.52
pH = 12.48
Example 4
If a solution has a pH of 7.41. What is its [H+], [OH–], pOH?
Solution
pH = 7.41
Recall,
pH = –log [H+]
7.41 = –log [H+]
–log [H+] = 7.41
log [H+] = –7.41
[H+] = Antilog –7.41
[H+] = 10–7.41
[H+] = 3.9 × 10–8 M
Recall,
[H+][OH–] = 1.0 × 10–14
3.9 × 10–8 × [OH–] = 1.0 × 10–14
[OH–] = \(\frac {1.0 × 10^{–14}}{3.9 × 10^{–8}}\)
[OH–] = 2.6 × 10–7 M
Recall,
pH + pOH = 14
7.41 + pOH = 14
pOH = 14 – 7.41
pOH = 6.59
[H+] = 3.9 × 10–8 M
[OH–] = 2.6 × 10–7 M
pOH = 6.59
Example 5
The pH of a solution of HCl is 2. The amount of acid present in a litre is...
(a) 2 g/L
(b) 3.65 g/L
(c) 0.365 g/L
(d) 36.5 g/L
Solution
This is a kind of question that requires you to understand it, before you attempt.
pH = 2
Volume = 1 L
We are to find Mass concentration = ?
From pH, we can get concentration of H+
pH = –log [H+]
2 = –log [H+]
–log [H+] = 2
log [H+] = –2
[H+] = 10–2 M
Equation to show dissociation of HCl is written as:
$$\small {\textnormal{HCl} \to \textnormal{H}^+ + \textnormal{Cl}^–}$$
1 mole of HCl produced 1 mole of H+
Therefore, 10–2 M of H+ is produced by 10–2 M of HCl.
Recall,
Mass concentration = Molar concentration × Molar mass
So, we still need to calculate for Molar mass of HCl
Molar mass of HCl:
= (1) + (35.5)
= 36.5 g/mol
We can then, finally calculate for mass concentration:
Mass concentration = 10–2 × 36.5
Mass concentration = 0.365 g/dm³
Option (c) is correct, 0.365 g/L.
Example 6
The concentration of H+ in a solution is 1.5 × 10–6 M. Find the concentration of OH– and state if the solution is acidic or basic.
Solution
[H+][OH–] = 1.0 × 10–14
1.5 × 10–6 × [OH–] = 1.0 × 10–14
[OH–] = \(\frac {1.0 × 10^{-14}}{1.5 × 10^{-6}}\)
[OH–] = 6.67 × 10–9 M
It is seen that [H+] > [OH–], hence, the solution is acidic.
Example 7
(a) Calculate the base dissociation constant, kb, for HBr given that ka = 6.8 × 10–4.
(b) Calculate the dissociation constant for nitrous oxide (kb = 2.0 × 10–11).
Solution
(a)
ka = 6.8 × 10–4
kb = ?
Recall,
ka × kb = kw
ka × kb = 1.0 × 10–14
6.8 × 10–4 × kb = 1.0 × 10–14
kb = \(\frac {1.0 × 10^{–14}}{6.8 × 10^{–4}}\)
kb = 1.5 × 10–11
(b)
kb = 2.0 × 10–11
ka = ?
Recall,
ka × kb = kw
ka × kb = 1.0 × 10–14
ka × 2.0 × 10–11 = 1.0 × 10–14
ka = \(\frac {1.0 × 10^{–14}}{2.0 × 10^{–11}}\)
ka = 5.0 × 10–4
Example 8
Vitamin C has a pKa value of 4.17, what are its ka and kb values ?
Solution
pKa = 4.17
ka = ?
kb = ?
Recall,
pKa = –log ka
4.17 = –log ka
–log ka = 4.17
log ka = – 4.17
ka = 10–4.17
ka = 6.76 × 10–5
Recall,
ka × kb = 1.0 × 10–14
6.76 × 10–5 × kb = 1.0 × 10–14
kb = \(\frac {1.0 × 10^{-14}}{6.76 × 10^{-5}}\)
kb = 1.48 × 10–10
Example 9
What is the pH of a 0.075 M Ba(OH)2 solution?
Solution
To get the pH of a basic solution, we need to first find [OH–]
Ba(OH)2 is a strong base. It dissociates completely in water to form:
$$\small { \textnormal{Ba(OH)}_2 \to \textnormal{Ba}^{2+} + 2\textnormal{OH}^–}$$
number of moles of OH– = 2
Then, [OH–] = 0.075 × 2
= 0.15 M
We calculate for pOH by:
pOH = –log [OH–]
pOH = –log 0.15
pOH = – (– 0.824)
pOH = 0.824
We finally calculate for pH by:
pH + pOH = 14
pH + 0.824 = 14
pH = 14 – 0.824
pH = 13.2
Example 10
If a solution has a pH of 5.5 at 25°C, what is its [OH–] ?
(a) 8.50 M
(b) 2.3 × 10–9 M
(c) 3.2 × 10–9 M
(d) 1.56 × 10–8 M
Solution
pH = 5.5
[OH–] = ?
To get [OH–], we shall calculate for pOH.
Recall,
pH + pOH = 14
5.5 + pOH = 14
pOH = 14 – 5.5
pOH = 8.5
We can then solve for [OH–]:
pOH = –log [OH–]
8.5 = – log [OH–]
–log [OH–] = 8.5
log [OH–] = – 8.5
[OH–] = 10–8.5
[OH–] = 3.2 × 10–9 M
Option (c) is correct.
Dissociation of weak acids and weak bases
Weak acids and weak bases do not dissociate completely in water, hence a solution of weak acids/bases contain both concentrations of the original acid/base and its dissociated ions.
The extent to which a weak acid/base ionizes in water depends on two factors which are:
1. the concentration of the original acid/base
2. the equilibrium constant for the ionization reaction; kafor acid or kb for base.
For an incomplete dissociation of a weak acid:
$$\small { \textnormal{HA} \rightleftharpoons \textnormal{H}^+ + \textnormal{A}^–}$$
The concentration of ions are shown in:
$$\small {\textnormal{k}_a = \frac {[\textnormal{H}^+][\textnormal{A}^-]}{[\textnormal{HA}]}}$$
Let us solve examples for you to see how to tackle these types of problems.
Example 1
Calculate the pH of a 0.50 M HF solution at 25°C? ka of HF = 7.1 x 10–4.
Solution
Original concentration of HF = 0.50 M
ka = 7.1 × 10–4
pH = ?
Since HF is a weak acid, it dissociates partially in water:
$$\small { \textnormal{HF} \rightleftharpoons \textnormal{H}^+ + \textnormal{F}^–}$$
To make our calculations easier, it is always better to quickly draw a short ICE table:
| HF | H+ | F– | |
| Initial | 0.50 | 0 | 0 |
| Change | –x | +x | +x |
| Equilibrium | 0.50 – x | x | x |
Recall,
$$\small { \textnormal{k}_a = \frac {[\textnormal{H}^+][\textnormal{F}^–]}{[\textnormal{HF}]}}$$
$$\small {7.1 × 10^{–4} = \frac {\textnormal{x × x}}{0.50 – \textnormal{x}}}$$
Because there's not enough time, and 0.50 is large, we usually put 0.50 – x ≈ 0.50
Hence, we have:
$$\small {7.1 × 10^{–4} = \frac {\textnormal{x}^2}{0.50}}$$
$$\small {7.1 × 10^{–4} \xcancel= \frac {\textnormal{x}^2}{0.50}}$$
$$\small {\textnormal{x}^2 = 7.1 × 10^{-4} × 0.50}$$
$$\small {\textnormal{x}^2 = 3.55 × 10^{-4}}$$
$$\small {\textnormal{x} = \sqrt{3.55 × 10^{-4}}}$$
$$\small {\textnormal{x} = 0.0188}$$
x = 0.0188 = concentration of Hydrogen ion [H+]
We can then go ahead to finally calculate for pH
pH = –log [H+]
pH = –log 0.0188
pH = –(–1.73)
pH = 1.73
Therefore, pH of 0.50 M of HF solution at 25°C is 1.73
Note:
There is something I want you to note concerning the above calculation.
We approximated 0.50 – x ≈ 0.50 so we would not get a quadratic equation that would result.
Hence, our previous answer is only very close to the real answer, but it is not the real answer.
The approximation method can only be carried out when initial concentration of the weak acid/base is large, like 0.5, 0.05. once we start having values like 0.0005, the quadratic method must be used.
To get the real answer for the above question, we would solve the quadratic equation step by step:
$$\small {7.1 × 10^{–4} = \frac {\textnormal{x × x}}{0.50 – \textnormal{x}}}$$
$$\small {7.1 × 10^{–4} \xcancel= \frac {\textnormal{x}^2}{0.50 – \textnormal{x}}}$$
$$\small {\textnormal{x}^2 = (7.1 × 10^{-4}) × (0.50 – \textnormal{x})}$$
$$\small {\textnormal{x}^2 = 0.000355\:\: – \:\: 7.1 × 10^{-4}\textnormal{x}}$$
$$\small {\textnormal{x}^2\:\: +\:\: 7.1 × 10^{-4}\textnormal{x}\:\: – \:\: 0.000355 \:\: = 0}$$
Using quadratic formula:
$$\small {\textnormal{x} = \frac{–b±\sqrt{b^2\: – \: 4ac}}{2a}}$$
$$\small {\textnormal{x} = \frac{–0.00071±\sqrt{0.00071^2 \: – \: 4 × 1 × –0.000355}}{2×1}}$$
$$\small {\textnormal{x} = \frac{–0.00071±\sqrt{5.04 × 10^{-7} + 0.00142}}{2}}$$
$$\small {\textnormal{x} = \frac{–0.00071±\sqrt{0.00142}}{2}}$$
$$\small { \textnormal{x} = \frac {-0.00071±0.038}{2}}$$
x cannot be negative, so we have:
$$\small { \textnormal{x} = \frac {-0.00071+0.038}{2}}$$
$$\small {\textnormal{x} = \frac {0.03729}{2}}$$
$$\small {\textnormal{x} = 0.0186}$$
You can see the process is a long one, and the final answer, 0.0186, is very close to our initial answer which was 0.0188.
pH of 0.0186 [H+] is 1.73 which is also close to our initial answer.
Example 2
Determine the pH for acetic acid solutions at 25°C with concentrations of:
(a) 0.15 M (b) 0.015 M
(ka = 1.8 × 10–5)
Solution
(a)
$$\small { \textnormal{CH}_3\textnormal{COOH} \rightleftharpoons \textnormal{CH}_3\textnormal{COO}^– + \textnormal{H}^+}$$
| CH3COOH | CH3COO– | H+ | |
| Initial | 0.15 | 0 | 0 |
| Change | –x | +x | +x |
| Equilibrium | 0.15 – x | x | x |
From:
$$\small {\textnormal{k}_a = \frac {[\textnormal{H}^+][\textnormal{CH}_3\textnormal{COO}^–]}{[\textnormal{CH}_3\textnormal{COOH}]}}$$
$$\small {1.8 × 10^{–5} = \frac {\textnormal{x × x}}{0.15 – \textnormal{x}}}$$
We put 0.15 – x ≈ 0.15
Hence, we have:
$$\small {1.8 × 10^{–5} = \frac {\textnormal{x}^2}{0.15}}$$
$$\small {1.8 × 10^{–5} \xcancel= \frac {\textnormal{x}^2}{0.15}}$$
$$\small {\textnormal{x}^2 = 1.8 × 10^{-5} × 0.15}$$
$$\small {\textnormal{x}^2 = 2.7 × 10^{-6}}$$
$$\small {\textnormal{x} = \sqrt{2.7 × 10^{-6}}}$$
$$\small {\textnormal{x} = 0.0016}$$
x = [H+] = 0.0016 M
Calculating for pH:
pH = –log [H+]
pH = –log 0.0016
pH = – (–2.796)
pH = 2.8
pH of 0.15 M acetic acid solution at 25°C is 2.8
(b)
| CH3COOH | CH3COO– | H+ | |
| Initial | 0.015 | 0 | 0 |
| Change | –x | +x | +x |
| Equilibrium | 0.015 – x | x | x |
From:
$$\small {\textnormal{k}_a = \frac {[\textnormal{H}^+][\textnormal{CH}_3\textnormal{COO}^–]}{[\textnormal{CH}_3\textnormal{COOH}]}}$$
$$\small {1.8 × 10^{–5} = \frac {\textnormal{x × x}}{0.015 – \textnormal{x}}}$$
We put 0.015 – x ≈ 0.015
Hence, we have:
$$\small {1.8 × 10^{–5} = \frac {\textnormal{x}^2}{0.015}}$$
$$\small {1.8 × 10^{–5} \xcancel= \frac {\textnormal{x}^2}{0.015}}$$
$$\small {\textnormal{x}^2 = 1.8 × 10^{-5} × 0.015}$$
$$\small {\textnormal{x}^2 = 2.7 × 10^{-7}}$$
$$\small {\textnormal{x} = \sqrt{2.7 × 10^{-7}}}$$
$$\small {\textnormal{x} = 0.00052}$$
x = [H+] = 0.00052 M
Calculating for pH:
pH = –log [H+]
pH = –log 0.00052
pH = – (–3.28)
pH = 3.28
pH of 0.015 M acetic acid solution at 25°C is 3.28
Example 3
Calculate the pH of 0.1 M NH3 solution.
(kb for NH3 = 1.8 × 10–5)
Solution
Ammonia is a weak base:
$$\small { \textnormal{NH}_3 + \textnormal{H}_2\textnormal{O} \rightleftharpoons \textnormal{NH}_4^+ + \textnormal{OH}^–}$$
| NH3 | NH4+ | OH– | |
| Initial | 0.1 | 0 | 0 |
| Change | –x | +x | +x |
| Equilibrium | 0.1 – x | x | x |
kb = \(\frac {\textnormal{x × x}}{0.1 – \textnormal{x}}\)
We put 0.1 – x ≈ 0.1
1.8 × 10–5 = \(\frac {\textnormal{x}^2}{0.1}\)
x2 = 0.1 × 1.8 × 10–5
x2 = 1.8 × 10–6
x = 0.0013416
x = [OH–] = 0.0013416 M
Calculating for pOH:
pOH = –log [OH–]
pOH = –log 0.0013416
pOH = –(–2.872)
pOH = 2.872
Calculating for pH:
pH + pOH = 14
pH = 14 – pOH
pH = 14 – 2.872
pH = 11.128
pH of 0.1 M ammonia solution is 11.128.
Example 4
Calculate the ka of a weak acid if a 0.015 M solution of the acid has a pH of 5.03 at 25°C.
Solution
pH = 5.03
Original concentration of acid = 0.015 M
ka = ?
$$\small{ \textnormal{HA} \rightleftharpoons \textnormal{H}^+ + \textnormal{A}^-}$$
We can calculate for [H+] from pH
pH = –log [H+]
5.03 = –log [H+]
H+ = 10–5.03
H+ = 9.3 × 10–6 M
| HA | H+ | A– | |
| Initial | 0.015 | 0 | 0 |
| Change | –9.3 × 10-6 | +9.3 × 10-6 | +9.3 × 10-6 |
| Equilibrium | 0.0149 | 9.3 × 10-6 | 9.3 × 10-6 |
Recall,
$$\small{ \textnormal{k}_a = \frac {[\textnormal{H}^+][\textnormal{A}^–]}{[\textnormal{HA}]}}$$
$$\small{ \textnormal{k}_a = \frac {9.3 × 10^{-6} × 9.3 × 10^{-6}}{0.0149}}$$
$$\small{ \textnormal{k}_a = 5.8 × 10^{-9}}$$
Example 5
Determine the kb of a weak base if a 0.35 M solution of the base has a pH of 11.84 at 25°C.
Solution
$$\small{ \textnormal{B} + \textnormal{H}_2\textnormal{O} \rightleftharpoons \textnormal{HB}^+ + \textnormal{OH}^-}$$
Since we are dealing with a base, we can find [OH–] from pH:
pH + pOH = 14
11.84 + pOH = 14
pOH = 14 – 11.84
pOH = 2.16
pOH = –log [OH–]
–log [OH–] = pOH
log [OH–] = –pOH
[OH–] = 10–pOH
[OH–] = 10–2.16
[OH–] = 0.00692
| B | HB+ | OH– | |
| Initial | 0.35 | 0 | 0 |
| Change | –0.00692 | +0.00692 | +0.00692 |
| Equilibrium | 0.34308 | 0.00692 | 0.00692 |
Recall,
$$\small{\textnormal{k}_b = \frac{[\textnormal{HB}^+][\textnormal{OH}^–]}{[\textnormal{B}]}}$$
We ignored [H2O] since it tends to unity
$$\small {\textnormal{k}_b = \frac {0.00692 × 0.00692}{0.34308}}$$
$$\small {\textnormal{k}_b = 1.4 × 10^{-14}}$$
Example 6
Calculate the pH at 25°C of a 0.16 M solution of a weak base with a kb of 2.9 × 10–11.
Solution
$$\small{ \textnormal{B} + \textnormal{H}_2\textnormal{O} \rightleftharpoons \textnormal{HB}^+ + \textnormal{OH}^–}$$
| B | HB+ | OH– | |
| Initial | 0.16 | 0 | 0 |
| Change | –x | +x | +x |
| Equilibrium | 0.16 – x | x | x |
Recall,
$$\small{\textnormal{k}_b = \frac{[\textnormal{HB}^+][\textnormal{OH}^–]}{[\textnormal{B}]}}$$
$$\small {\textnormal{k}_b = \frac {\textnormal{x} × \textnormal{x}}{0.16 – \textnormal{x}}}$$
We put 0.16 – x ≈ 0.16
$$\small {2.9 × 10^{-11} = \frac { \textnormal{x}^2}{0.16}}$$
$$\small{ \textnormal{x}^2 = 2.9 × 10^{–11} × 0.16}$$
$$\small{\textnormal{x}^2 = 4.64 × 10^{-12}}$$
$$\small{\textnormal{x} = 2.15 × 10^{-6}}$$
Thus;
[OH–] = 2.15 × 10-6 M
We can calculate for pOH by:
pOH = –log [OH–]
pOH = –log 2.15 × 10–6
pOH = – (–5.67)
pOH = 5.67
Recall,
pH + pOH = 14
pH = 14 – pOH
pH = 14 – 5.67
pH = 8.33
Indicators
To identify whether a substance or solution is acidic or basic, indicators are used.
Indicators are weak organic substances that change colour in the presence of an acid or base.
Acid–base indicators change colour when hydroxonium or hydroxide ion concentration is changed.
There are two common indicators:
Universal indicators and pH meter.
Universal Indicator
Some indicators are used as mixtures. The mixture indicator gives different colours at different pH values. Hence, it is used to measure the pH of a solution. Such a mixed indicator is called Universal Indicator or simply pH indicator. The pH of solution can be measured by dipping a piece of Universal Indicator paper, or dropping small quantities of liquid in the solution. The pH is then found by comparing the colour obtained with a general colour chart depending on the type of indicator.
| Indicator | Colour in acids | pH range at which colour changes | Colour in bases |
|---|---|---|---|
| Methyl Orange | Pink | 3.1 – 4.4 | Yellow |
| Methyl Red | Red | 4.4 – 6.2 | Yellow |
| Bromothymol Blue | Yellow | 6.0 – 7.6 | Blue |
| Phenolphthalein | Colourless | 8 – 9 | Pink |
| Litmus | Red | 4.5 – 8.5 | Blue |
| Bromocresol Green | Yellow | 3.8 – 5.4 | Blue |
| Thymol Blue | Yellow | 8.0 – 9.6 | blue |
pH meter
The pH of a solution can be measured with a pH meter. It consists of a pH electrode connected to a meter. The electrode is dipped into the solution and the meter shows the pH either on a scale or digitally. It is a much more reliable and accurate method of measuring pH than Universal Indicator, though the latter is often more convenient.
pH scale
Buffers and Henderson–Hasselbalch Equation
A buffer is a solution containing a weak acid (or weak base) and a salt of the same weak acid (or weak base).
An example of a buffer solution is an aqueous mixture of acetic acid and sodium acetate (CH3COOH and CH3COONa). A mixture of acetic acid and sodium chloride is NOT a buffer solution (because NaCl is not a salt of CH3COOH).
Buffer solutions have the ability to resist pH changes when small amounts of strong acids or bases are added to the solution.
pH of a buffer solution is estimated by the Henderson–Hasselbalch equation:
$$\textnormal{pH} = \textnormal{pK}_a \:\: + \:\: log\frac {[\textnormal{A}^–]}{[\textnormal{HA}]}$$
Where [A–] is the concentration of the conjugate base of the acid in the salt.
[HA] is the concentration of the acid.
pKa = –log ka
For buffer solutions containing a weak Monoprotic acid and one of its salt, Henderson–Hasselbalch equation can be quickly written as:
$$\textnormal{pH} = \textnormal{pK}_a \:\: + \:\: log\frac {[\textnormal{salt}]}{[\textnormal{acid}]}$$
But in a standard manner, Henderson–Hasselbalch equation is written as:
$$\textnormal{pH} = \textnormal{pK}_a \:\: + \:\: log\frac {[\textnormal{conjugate base}]}{[\textnormal{acid}]}$$
$$\textnormal{pOH} = \textnormal{pK}_b \:\: + \:\: log\frac {[\textnormal{conjugate acid}]}{[\textnormal{base}]}$$
Example 1
Calculate the pH of a solution containing 0.0500 M acetic acid and 0.0250 M sodium acetate. (The ka of acetic acid is 1.8 x 10–5)
Solution
concentration of acetic acid, [HA], = 0.0500 M
concentration of the conjugate base of acetic acid (CH3COO–) in sodium acetate, [A–], is:
$$\small {\textnormal{CH}_3\textnormal{COONa} \rightleftharpoons \textnormal{CH}_3\textnormal{COO}^– + \textnormal{Na}^+}$$
1 M CH3COONa yield 1 M CH3COO–
0.0250 M CH3COONa will yield 0.0250 M CH3COO–
Thus,
[A–] = 0.0250 M
ka = 1.8 × 10–5
Recall Henderson–Hasselbalch equation,
$$\textnormal{pH} = \textnormal{pK}_a \:\: + \:\: log\frac {[\textnormal{A}^–]}{[\textnormal{HA}]}$$
We need to solve for pKa:
pKa = –log ka
pKa = –log 1.8 × 10–5
pKa = –(– 4.74)
pKa = 4.74
We can then solve for pH of the buffer solution:
= 4.74 + log\(\frac{0.0250}{0.0500}\)
= 4.74 + log 0.5
= 4.74 + (–0.30)
= 4.74 – 0.30
= 4.44
pH of of a solution containing 0.0500 M acetic acid and 0.0250 M sodium acetate is 4.44
Example 2
A buffer solution contains 0.5 M NH3 and 0.8 M NH4Cl. Calculate the pH of the solution.
(kb = 1.8 × 10–5)
Solution
pH = ?
kb = 1.8 × 10–5
[B] = 0.5
[HB+] = 0.8
pKb = –log kb
pKb = –log 1.8 × 10–5
pKb = – (– 4.74)
pKb = 4.74
We can then calculate for pOH:
\(\small{\textnormal{pOH} = \textnormal{pK}_b} + log \frac{[\textnormal{HB}^+]}{[\textnormal{B}]}\)
\(\small{\textnormal{pOH} = 4.74} + log \frac{0.8}{0.5}\)
\(\small{\textnormal{pOH} = 4.74 + log 1.6}\)
\(\small{\textnormal{pOH} = 4.74 + 0.2}\)
\(\small{\textnormal{pOH} = 4.94}\)
Recall,
pH + pOH = 14
pH + 4.94 = 14
pH = 14 – 4.94
pH = 9.06
The pH of a buffer solution that contains 0.5 M NH3 and 0.8 M NH4Cl is 9.06
Example 3
Calculate pH of a buffer solution that is 0.25 M in Formic acid and 0.1 M in Sodium formate.
(ka for formic acid is 1.8 × 10–4)
Solution
pH = ?
[HA] = 0.25
[A–] = 0.1
ka = 1.8 × 10–4
pKa = –log ka
pKa = –log 1.8 × 10–4
pKa = 3.74
Recall,
\(\small{\textnormal{pH} = \textnormal{pK}_a} + log \frac{[\textnormal{A}^–]}{[\textnormal{HA}]}\)
\(\small{\textnormal{pH} = 3.74} + log \frac{0.1}{0.25}\)
\(\small{\textnormal{pH} = 3.74 + log 0.4}\)
\(\small{\textnormal{pH} = 3.74 – 0.4}\)
\(\small{\textnormal{pH} = 3.34}\)
pH of a buffer solution containing 0.25 M formic acid and 0.1 M sodium formate is 3.34
Example 4
The pH of a solution at 25°C is 5.27 and it contains 0.1 M of sodium acetate. What is the concentration of the acetic acid.
(pKa for CH3COOH is 4.75)
Solution
pH = 5.27
[A–] = 0.1
[HA] = ?
pKa = 4.75
Recall,
\(\small{\textnormal{pH} = \textnormal{pK}_a} + log \frac{[\textnormal{A}^–]}{[\textnormal{HA}]}\)
\(\small{5.27 = 4.75} + log \frac{0.1}{[\textnormal{HA}]}\)
\(\small{ 5.27 – 4.75 = }log \frac{0.1}{[\textnormal{HA}]}\)
\(\small{ 0.52 = }log \frac{0.1}{[\textnormal{HA}]}\)
\(\log \frac{0.1}{[\textnormal{HA}]} = \small{0.52}\)
\(\frac {0.1}{[\textnormal{HA}]} = \small { 10^{0.52}}\)
\(\frac {0.1}{[\textnormal{HA}]} = \small {3.31}\)
\(\small{[\textnormal{HA}]} = \frac {0.1}{3.31}\)
\(\small{[\textnormal{HA}]} = \small {0.03}\)
Concentration of acetic acid in the given buffer solution is 0.03 M
Example 5
What is the pH of a buffer that is 0.12 M in lactic acid [HC3H5O3] and 0.10 M in sodium lactate [NaC3H5O3]?
Given that ka (lactic acid) = 1.4 × 10–4
Solution
[HA] = 0.12 M
[A–] = 0.10 M
ka = 1.4 × 10–4
Recall,
\(\small{\textnormal{pH} = \textnormal{pK}_a} + log \frac{[\textnormal{A}^–]}{[\textnormal{HA}]}\)
But we need pKa;
pKa = –log ka
pKa = –log 1.4 × 10–4
pKa = 3.85
\(\small{\textnormal{pH} = 3.85} + log \frac{0.10}{0.12}\)
\(\small{ \textnormal{pH} = 3.85 + log 0.833}\)
\(\small{ \textnormal{pH} = 3.85 – 0.079}\)
\(\small{ \textnormal{pH} = 3.771}\)
Example 6
A solution at 25°C contains 0.10 M sodium acetate and 0.03 M acetic acid. What is its pH?
(pKa for CH3COOH is 4.75).
Solution
[A–] = 0.10 M
[HA] = 0.03 M
pH = ?
pKa = 4.75
Recall,
\(\small{\textnormal{pH} = \textnormal{pK}_a} + log \frac{[\textnormal{A}^–]}{[\textnormal{HA}]}\)
\(\small{\textnormal{pH} = 4.75} + log \frac{0.10}{0.03}\)
\(\small{\textnormal{pH} = 4.75 + log 3.3333}\)
\(\small {\textnormal{pH} = 4.75 + 0.52}\)
\(\small{ \textnormal{pH} = 5.27}\)
A solution containing 0.10 M sodium acetate and 0.03 acetic acid has a pH of 5.27 at 25°C.
Example 7
Calculate the concentration of sodium formate that must be present in a 0.10 M solution of formic acid to produce a pH of 3.80.
(ka for formic acid = 1.8 × 10–4)
Solution
[A–] = ?
[HA] = 0.10 M
pH = 3.80
ka = 1.8 × 10–4
Recall,
\(\small{\textnormal{pH} = \textnormal{pK}_a} + log \frac{[\textnormal{A}^–]}{[\textnormal{HA}]}\)
We need pKa;
pKa = –log ka
pKa = –log 1.8 × 10–4
pKa = 3.74
\(\small{3.80 = 3.74} + log \frac{[\textnormal{A}^–]}{0.10}\)
\(log \frac{[\textnormal{A}^–]}{0.10} = \small{3.80 – 3.74}\)
\(log \frac{[\textnormal{A}^–]}{0.10} = \small{0.06}\)
\(\frac {[\textnormal{A}^–]}{0.10} = \small {10^{0.06}}\)
\(\frac {[\textnormal{A}^–]}{0.10} = \small {1.148}\)
\(\small {[\textnormal{A}^–] = 0.115}\)
A solution that contains 0.10 M of formic acid must have a 0.115 M concentration of sodium formate to produce a pH of 3.80.
Note:
For a Monoprotic acid (HA),
Percent Ionization = \(\frac{[\textnormal{H}^+]_{eq}}{[\textnormal{HA}]_{initial}} × \small {100 \%}\)
I would advise that you read this post more than once, while trying to also attempt the examples by yourself. Don't be scared to meet any senior level student for a better explanation on whatever it is that you do not understand. I wish you the best.
Summary
📌 Arrhenius defined acids as substances which dissociate in water to give H+ ions, while bases are substances that dissociate in water to give OH– ions.
📌 Brønsted–Lowry defined acids as any substance that is capable of donating a proton, while bases are substances that are capable of accepting a proton.
📌 Lewis defined acids as substances that can accept paired electrons, while bases are substances that can donate electron pairs.
📌 Lewis acid + Lewis base = Adduct.
📌 What remains after an acid has lost a proton is Conjugate base.
📌 What is formed after a base accepts a proton is Conjugate acid.
📌 Strong acids and bases ionise completely in water. Weak acids and bases do not.
📌 Salts of:
- Strong acid + Strong base = Neutral solution
- Strong acid + Weak base = Acidic solution
- Weak acid + Strong base = Basic solution
- Weak acid + Weak base = More info required
📌 Amphoteric substances are substances that can act as an acid, and also a base.
📌 Amphiprotic substances are substances that can donate protons and also accept protons.
📌 Water undergo self dissociation to a very small degree, called autoprotolysis.
📌 pH of buffer solutions are resistant to addition of small amounts of acids or bases.
Key Terms
Hydrogen ions (H+) • Hydroxide ions (OH–) • Dissociation • Ionisation • Conjugate base • Conjugate acid • Conjugate acid–base pair • Molecular acids • Anion acids • Cation acids • Molecular base • Anion base • Cation base • Protonic solvents • Non–protonic solvents • Lewis acids • Lewis bases • Adduct • Co-ordination Complex compound • Co-ordinate covalent bond • Dative bond • Hard acids • Hard bases • Soft acids • Soft bases • Borderline acids • Borderline bases • Corrosive • Strong acids • Weak acids • Basicity • Monoprotic • Diprotic • Polyprotic • Equilibrium reaction • Strong bases • Weak bases • Salts • Simple salts • Double salts • Mixed salts • Complex salts • Acidic salts • Basic salts • Hydrolysis • Amphoteric • Amphiprotic • Mass concentration • Molar concentration • Autoprotolysis • Equilibrium constant • Activity • Dissociation constant • Potential • Universal indicators • pH meter • Buffer solutions • Concentration of hydrogen ion [H+] / [H3O+] (hydroxonium ion concentration) • Concentration of hydroxide ion [OH–] • ka • kb • kw • pH • pOH • pKa • pKb • Concentration of conjugate base [A–] • Concentration of conjugate acid [HB+]
Recommended Videos
Test Questions
Discuss And Explain
1. Explain Lewis concept of acids and bases.
2. Define an acid and a base according to Brønsted–Lowry concept and justify with equations that water is amphoteric.
3. What is auto–ionisation of water? How is it used to establish the pH of water?
4. Explain why:
- HCl forms only one series of salts.
- H2SO4 forms two series of salts.
- H3PO4 forms three series of salts.
5. State why stains of curry on a white cloth becomes reddish brown when soap is scrubbed on it, and turns yellow again when the cloth is washed in plenty of water.
6. An unknown gas reacts with lime water and form a compound which is used as a bleaching agent in chemistry. Identify the unknown gas and unknown compound.
7. Name the compound which is obtained from baking soda and is used to remove permanent hardness of water. Write its chemical formula, what type of salt it is, and state whether its aqueous solution will be acidic or alkaline.
8. Name the products formed in each of these reactions:
- Hydrochloric acid reacts with caustic soda.
- Granulated zinc reacts with caustic soda.
- Carbon(IV) oxide is passed into lime water.
9. What is an alkali? Give three examples.
10. Differentiate Amphoteric and Amphiprotic.
11. Describe the formation of adducts by Lewis acids and Lewis bases.
12. How is hydrogen ion concentration and pH related to each other? Explain.
13. Give the chemical formulas of washing soda and baking soda. Describe how washing soda is manufactured by solvay's method?
14. List any four uses of Plaster of Paris.
15. What is bleaching? Chemically, what is bleaching powder? Give its any four uses.
16. What is the importance of pH for humans and animals and our digestive system? Explain what you understand by the term universal indicator.
17. What is pH? What happens to the pH of a solution if the hydroxyl ion ion concentration increases?
18. What is self–dissociation of water. Give the resulting species and their concentrations at 25°C. Will the value change if an acid, base, or salt is dissolved in water?
19. What is the pH of 1.0 × 10–5 molar solution of KOH?
20. What is the pH of 1.0 × 10–2 mol/L solution of NaCl?






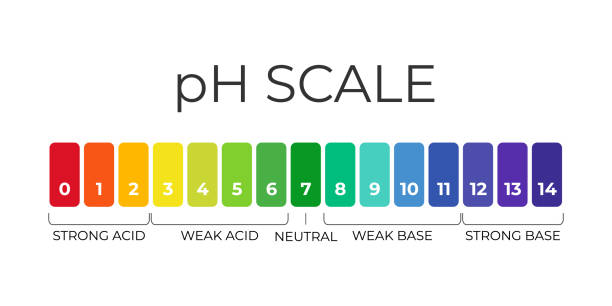


![GROUP 3A ELEMENTS [BORON FAMILY]](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEiZDc2JthEzvVH7OrNyvSOxTX8pU5a4B_TFZlizMt4xjbWXHPyUiINyQdUvBc1Hz2oa82Ai0q5jmzB3DMPa9c5KWV5PIm-lDdHVB8P9WjJopbFFfUb56HaQbi-fb3A1jCnUBQs9gXvjFMH9HsXWpk8W7mVOv8qwfjTmg_h50R0S0JEKwNXd1hPpiMD2gA/w74-h74-p-k-no-nu/Group%2013%20or%20Group%203A%20elements.jpg)

![GENERAL QUESTIONS ON ANP 101 [MEDIUM]](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEh3Jdd42xekrYhc5OIwQ7vWl-_Qy9jM6WrMuuU8dhKeBulL5_YFhTnSfYfV1e6sTp2-TKsqVDbs4IJGIg1MgmOLSLqP5VfTbZ37VWa2cvRFXzezWRXRJkNnLuMpNDBKDBLbVjOG-F7uq8d-Yst4T4tZ8_fEvLG2bLenjuL0NuEbtrQuiJ_nznG3eDu0XQ/w74-h74-p-k-no-nu/20220704_063500.png)



![GROUP 2A ELEMENTS [ALKALINE EARTH METALS]](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEhUTYpUkiQFbILel9JPRlgAwakjh5TkZ8Fy_UeC3eywCC44gUow-vUGepTfcCf_ugBdIzDRIWApEkx7Nzw5qELf5O70Wm1nvjgZLjsFNjogyLtTLqWY3Wevv1Fx725f4KQpc_cO5y7x_2tWXK2RdBKGOkEK05UsIaKSleYavjWXqtydoYizp_8a4VkliA/w74-h74-p-k-no-nu/Group%202%20elements%20alkaline%20earth%20metals.jpg)


